
A car is moving on a circular level road of curvature 300m. If the coefficient of friction is 0.3 and acceleration due to gravity is
A. 30km/h
B. 81km/h
C. 108 km/h
D. 162 km/h
Answer
509.4k+ views
Hint: Use the concept of banking of road. Try to resolve the forces on the car. Gravitational force and normal force will definitely be on the car which is balancing each other. In this question, if a car is slipping away from the road then centrifugal force must be acting on the body. We know that maximum speed is given by following formula
Complete step-by-step answer:
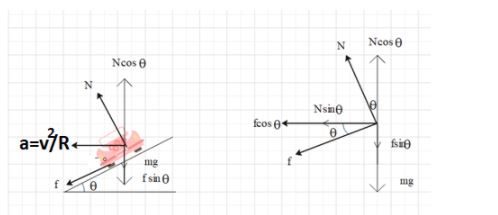
Consider a vehicle of mass
(1) Weight mg acting vertically downward (2) Normal reaction
The frictional force between tyres of vehicle and road surface can be resolved into
The normal reaction can be resolved into two components,
The component
Horizontal component
Dividing (1) by (2), we get
The magnitude of frictional force depends on the speed of the vehicle for the given road surface and tyres of the vehicle.
Let
For a curved horizontal road
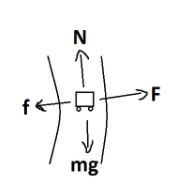
Aim- When a car is turning from the circular road then a car will sleep if it exceeds maximum speed. Find its maximum speed.
Force acting on the car are:
Force acting downward on the car which is mg
To balance this force, a normal force acts i.e. N
Outside of the car, force acting is centrifugal force F
And to balance this centrifugal force, a frictional force is acting toward the road as shown in the diagram.
So from this above statement, we can write as
N=mg -------(1)
And
We know that centrifugal force is the force acting away from the centre of the body when the body does circular motion and it is given as,
To balance the car and make a smooth turn while running the car on a curved road, frictional force must be equal to centripetal force.
From equation (1) and (2),
Cancel m from both sides.
We get,
The calculated value of velocity is in m/s but our options are in km/s. So convert m/s into km/s.
A maximum speed of car can be
So, the correct answer is “Option C”.
Note: Do not get confused between centripetal force and Centrifugal force. Centrifugal force is the force acting away from the centre of the body when the body does circular motion. Centripetal force is the force acting towards the centre of the body when the body does circular motion. Look for units. Once calculation has been done, check options in which unit it is given. Convert it accordingly. To convert m/s into km/s, multiply answer by 18 and divide it by 5.
Complete step-by-step answer:

Consider a vehicle of mass
(1) Weight mg acting vertically downward (2) Normal reaction
The frictional force between tyres of vehicle and road surface can be resolved into
The normal reaction can be resolved into two components,
The component
Horizontal component
Dividing (1) by (2), we get
The magnitude of frictional force depends on the speed of the vehicle for the given road surface and tyres of the vehicle.
Let
For a curved horizontal road

Aim- When a car is turning from the circular road then a car will sleep if it exceeds maximum speed. Find its maximum speed.
Force acting on the car are:
Force acting downward on the car which is mg
To balance this force, a normal force acts i.e. N
Outside of the car, force acting is centrifugal force F
And to balance this centrifugal force, a frictional force is acting toward the road as shown in the diagram.
So from this above statement, we can write as
N=mg -------(1)
And
We know that centrifugal force is the force acting away from the centre of the body when the body does circular motion and it is given as,
To balance the car and make a smooth turn while running the car on a curved road, frictional force must be equal to centripetal force.
From equation (1) and (2),
Cancel m from both sides.
We get,
The calculated value of velocity is in m/s but our options are in km/s. So convert m/s into km/s.
A maximum speed of car can be
So, the correct answer is “Option C”.
Note: Do not get confused between centripetal force and Centrifugal force. Centrifugal force is the force acting away from the centre of the body when the body does circular motion. Centripetal force is the force acting towards the centre of the body when the body does circular motion. Look for units. Once calculation has been done, check options in which unit it is given. Convert it accordingly. To convert m/s into km/s, multiply answer by 18 and divide it by 5.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




