Question

A car when passes through a convex bridge exert a force on it which equals to:
A. $Mg + \dfrac{{M{v^2}}}{r}$
B. $\dfrac{{M{v^2}}}{r}$
C. $Mg - \dfrac{{M{v^2}}}{r}$
D. None of the above

Answer 1

Hint: For a car moving on a curved bridge, the car will exert the normal force on the bridge as it moves through it. The weight of the car will be acting downwards, opposite to the normal reaction. Then the difference of the weight and normal reaction will provide the necessary centripetal force to the car.

Complete step by step solution:
Let r be the radius of the convex bridge and let us now draw the free body diagram of the car on the bridge.
Following is the diagram showing the forces acting on the car.

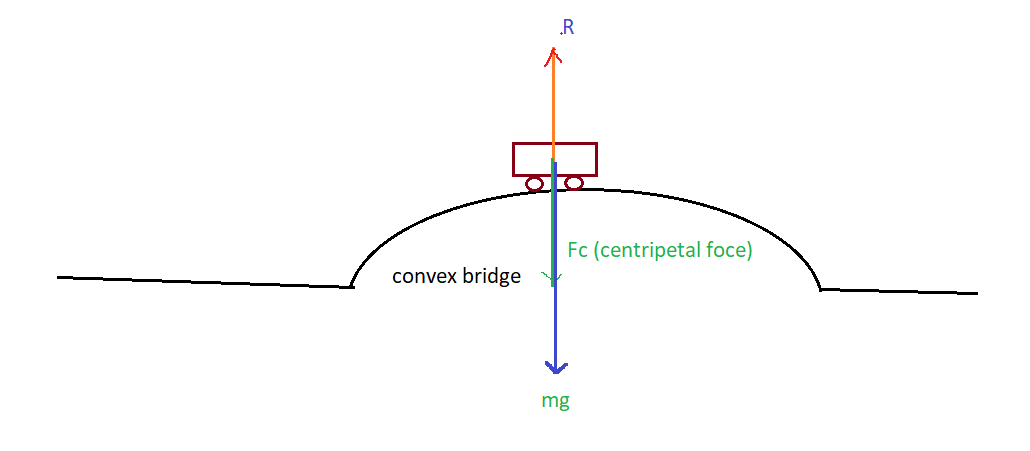
We can write from the diagram.
$Mg - {F_c} = R$ ………………….(1)
Here, ${F_c}$ is the centripetal force, $M$ is the mass of the car, $g$ is the acceleration due to gravity, and $R$ is the normal reaction.
The centripetal force is given by the following formula.
${F_c} = \dfrac{{M{v^2}}}{r}$
Here, $r$ is the radius of the convex bridge and $v$ is the speed of the car.
Putting this value in equation (1), we get the following expression.
$Mg - \dfrac{{M{v^2}}}{r} = R$
Therefore, the force exerted by the car when it passes through a curved bridge is given below.
$Mg - \dfrac{{M{v^2}}}{r} = F$
Hence, the correct option is (C) $Mg - \dfrac{{M{v^2}}}{r}$.

Note:
The force exerted by the car when it passes through the concave bridge is given below.
$Mg + \dfrac{{M{v^2}}}{r} = F$
A body doing circular motion is always acted by a force called centripetal force and the direction of this force is always towards the center.
As the radius of the curved path decreases the magnitude of centripetal force increases.