
A charged particle enters a magnetic field at right angles to the field. The field exists for a length equal to
A.
B.
C.
D.
Answer
478.8k+ views
1 likes
Hint: We are given that the particle enters the magnetic field at right angle. The particle traces a circular path. The length of the field exceeds the radius of the circular path of the particle. We need to find the deviation of the particle from the circular path. If the length of the magnetic field is more than the radius of the circular path then the particle exits the magnetic field from the same side as it enters.
Complete step by step answer:
The force
The direction of the force is given by the cross product between the velocity and the magnetic field.
Let us draw an appropriate diagram for the same.
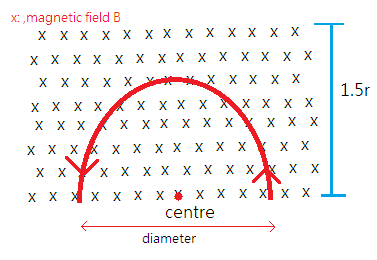
It is clear from the particle enter from the right-hand side and exits from the left-hand side. The length of the magnetic field is
For a length greater than the radius of the circular path traced by the particle, the particle exits from the same side from which it enters. Hence, the angle of deviation for the particle is
So, the correct answer is “Option D”.
Note:
For the particle to reach the maximum displacement the field must have a minimum length which is equal to the radius of the circular path traced by the particle.
The direction force acting on the particle is perpendicular to the direction of the magnetic field is given by the cross product between velocity and magnetic field.
Complete step by step answer:
The force
The direction of the force is given by the cross product between the velocity and the magnetic field.
Let us draw an appropriate diagram for the same.

It is clear from the particle enter from the right-hand side and exits from the left-hand side. The length of the magnetic field is
For a length greater than the radius of the circular path traced by the particle, the particle exits from the same side from which it enters. Hence, the angle of deviation for the particle is
So, the correct answer is “Option D”.
Note:
For the particle to reach the maximum displacement the field must have a minimum length which is equal to the radius of the circular path traced by the particle.
The direction force acting on the particle is perpendicular to the direction of the magnetic field is given by the cross product between velocity and magnetic field.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Earth rotates in which direction A East to west B West class 11 physics CBSE

Difference between physical and chemical change class 11 chemistry CBSE

Whales are warmblooded animals which live in cold seas class 11 biology CBSE




