
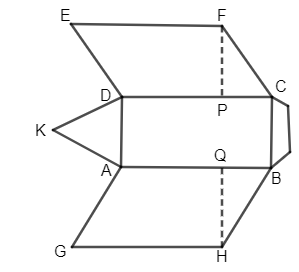
A child draws the figure of an aeroplane as given. Here the wings EDCF and AGHB are parallelograms, the tail ADK is an isosceles triangle, the cockpit BLC is a semicircle and the portion ABCD is a square. Let
(a)
(b)
(c)
(d)

Answer
505.5k+ views
Hint: To find the total area of the aeroplane as per the drawing, we will find the area of parts in which the figure is divided such as, area of square, area of parallelogram, area of semicircle, and area of triangle. Then we will sum up all the areas and we will get the total area.
Complete step-by-step answer:
In question it is given that a child draws an aeroplane which can be seen in figure below,
The plane is made by using different shapes such as, square, triangle, parallelogram and semi-circle. And we are asked to find the total area of the figure. So, to find total area we will divide the figure in different shapes as per the figure itself and then we will find the areas such as,
Where, s is semi perimeter of three sides and a, b and c are the sides of triangle
Now, first of all we will consider the parallelograms, EDCF and AGHB, so the area of parallelograms EDCF and AGHB using the expression (iii) can be given as,
Now, parallelograms EDCF and AGHB are same in size so, we can say that
Now, for parallelogram AGHB breadth is AB and altitude is HQ, and their values are
Now, on considering the triangle ADK, the area of triangle can be given by using the formula (i) which can be seen as,
Now, s can be given as,
Now, substituting these values of s in expression of triangle and substituting values of a, b and c, i.e. 6, 5, and 5 we will get,
Now, on considering square ABCD, area of square can be given as,
Now, the value of side AB is
Now, on considering semicircle BLC, area of semicircle can be given as,
Now, from the figure we can see that the radius of the semicircle is half the length of side BC, so the value of radius will be
No, total area is summation of all the areas which can be given as,
So, substituting all the values we will get,
Hence, the total area of figure is
Option (c) is the correct answer.
Note: Students must know all the formulas which are used in this problem. Otherwise the answer will be incorrect. Also, area of triangle can be found out by drawing perpendicular bisector i.e. KM such that triangle KML and KMD will be right angle So, KM and MD will be 3cm. Using Pythagora's theorem, we can find length of KM which will be equal to 4. So, then we have two right angle triangles. So, using the formula
Complete step-by-step answer:
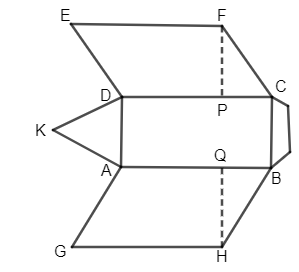
In question it is given that a child draws an aeroplane which can be seen in figure below,

The plane is made by using different shapes such as, square, triangle, parallelogram and semi-circle. And we are asked to find the total area of the figure. So, to find total area we will divide the figure in different shapes as per the figure itself and then we will find the areas such as,
Where, s is semi perimeter of three sides and a, b and c are the sides of triangle
Now, first of all we will consider the parallelograms, EDCF and AGHB, so the area of parallelograms EDCF and AGHB using the expression (iii) can be given as,
Now, parallelograms EDCF and AGHB are same in size so, we can say that
Now, for parallelogram AGHB breadth is AB and altitude is HQ, and their values are
Now, on considering the triangle ADK, the area of triangle can be given by using the formula (i) which can be seen as,
Now, s can be given as,
Now, substituting these values of s in expression of triangle and substituting values of a, b and c, i.e. 6, 5, and 5 we will get,
Now, on considering square ABCD, area of square can be given as,
Now, the value of side AB is
Now, on considering semicircle BLC, area of semicircle can be given as,
Now, from the figure we can see that the radius of the semicircle is half the length of side BC, so the value of radius will be
No, total area is summation of all the areas which can be given as,
So, substituting all the values we will get,
Hence, the total area of figure is
Option (c) is the correct answer.
Note: Students must know all the formulas which are used in this problem. Otherwise the answer will be incorrect. Also, area of triangle can be found out by drawing perpendicular bisector i.e. KM such that triangle KML and KMD will be right angle So, KM and MD will be 3cm. Using Pythagora's theorem, we can find length of KM which will be equal to 4. So, then we have two right angle triangles. So, using the formula
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




