
A chord of a parabola cuts the axis of the parabola at O. The feet of the perpendiculars from P and P’ on the axis are M and M’ respectively. If V is the vertex then VM, VO, VM’ are
(a) A.P
(b) G.P
(c) H.P
(d) AP, GP
Answer
492.9k+ views
Hint: To solve this question we will first take an equation of a parabola and then try to draw its figure using the given points in the question. Any point P on the parabola is of the form
Complete step-by-step answer:
To solve this question, we will first consider some parabola. To do that let us define a parabola and some examples. The parabola is the locus of points in that plane that are equidistant from both the directrix and the focus. Another description of a parabola is a conic section, created from the intersection of a right circular conical surface and a plane parallel to another plane that is tangential to the conical surface. The examples of the standard parabola are:
It is drawn as
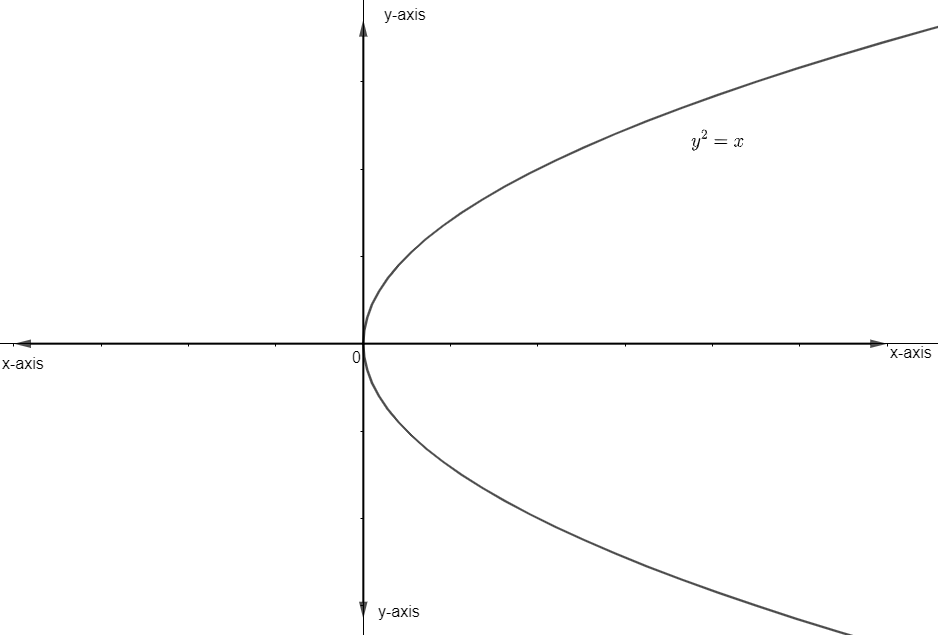
It is drawn as
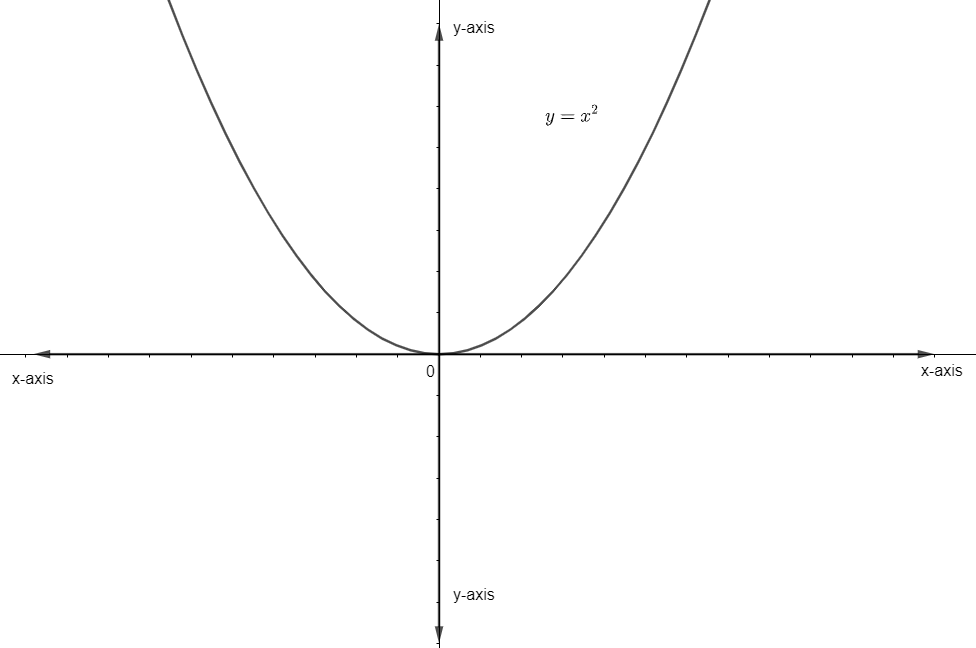
From these, let the parabola be
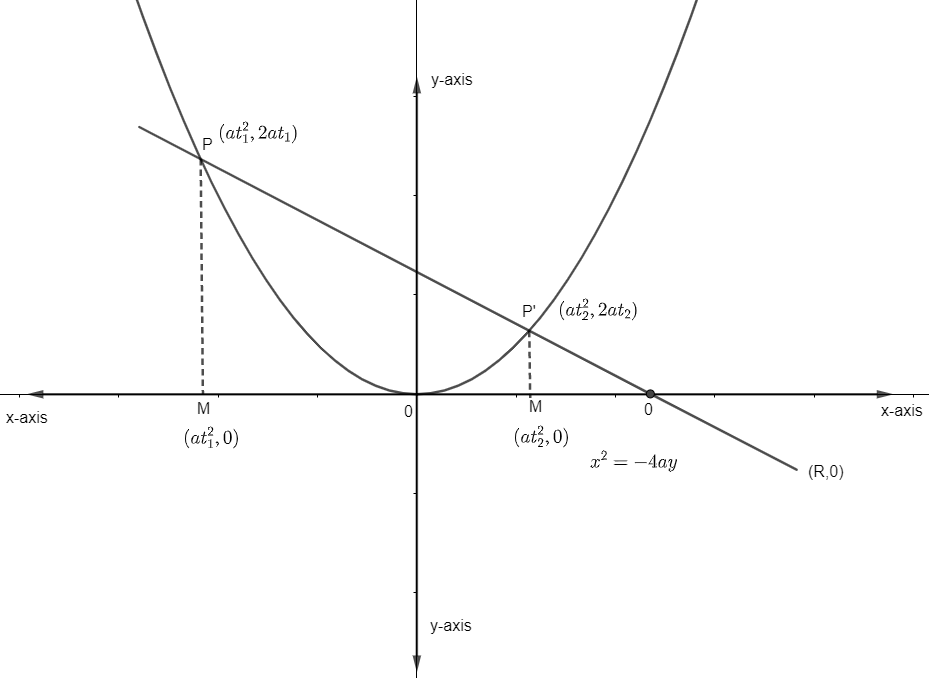
Let the vertex of parabola be V = (0, 0). The chord PP’ cuts x-axis at 0 and let it be (R, 0).
Let
Similarly, the slope of P’O is given by (using the above formula) the slope of
Now because PO and P’O are forming the same line, so then the slopes are equal.
The slope of PO = Slope of P’O
On cross multiplying, we get,
Hence, the value of R is
So, we have,
Substituting
Hence, VM, VO, VM’ are in GP.
So, the correct answer is “Option (c)”.
Note: When three numbers a, b and c are in GP, then they can be written as
Complete step-by-step answer:
To solve this question, we will first consider some parabola. To do that let us define a parabola and some examples. The parabola is the locus of points in that plane that are equidistant from both the directrix and the focus. Another description of a parabola is a conic section, created from the intersection of a right circular conical surface and a plane parallel to another plane that is tangential to the conical surface. The examples of the standard parabola are:
It is drawn as

It is drawn as

From these, let the parabola be

Let the vertex of parabola be V = (0, 0). The chord PP’ cuts x-axis at 0 and let it be (R, 0).
Let
Similarly, the slope of P’O is given by (using the above formula) the slope of
Now because PO and P’O are forming the same line, so then the slopes are equal.
The slope of PO = Slope of P’O
On cross multiplying, we get,
Hence, the value of R is
So, we have,
Substituting
Hence, VM, VO, VM’ are in GP.
So, the correct answer is “Option (c)”.
Note: When three numbers a, b and c are in GP, then they can be written as
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




