
A circle is described whose center is the vertex and whose diameter is three-quarters of the latus rectum of a parabola. Prove that the common chord of the circle and parabola bisects the distance between the vertex and the focus.
Answer
510k+ views
Hint: Draw a suitable diagram with the help of given information to visualize the problem accurately. Latus rectum of parabola
Complete step-by-step answer:
Let us suppose the equation of parabola be
So, diagram of parabola is given as
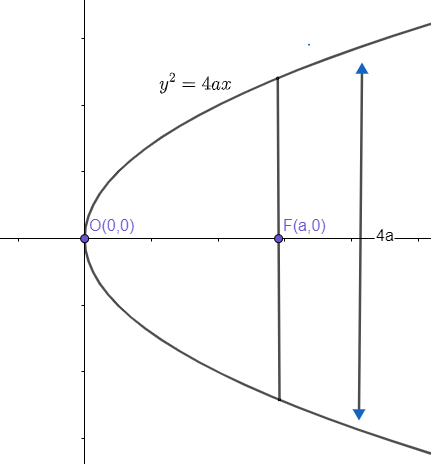
Where (a, o) is the focus of the parabola.
Now, a circle is drawn with center at the vertex i.e. at (o, o) and diameter of
Now, we know the standard equation of the circle is given by equation
Where,
So, equation of the circle with (0, 0) as centre and radius as
Now, we have to prove that the common chord of the circle and parabola bisects the distance between vertex and focus.
So, diagram with the help of above information is given as:
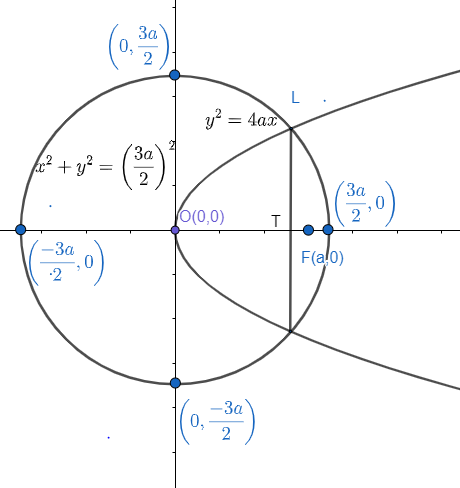
Where line L is the common chord of parabola and circle, as given in the diagram and F(a, 0) is the focus of the parabola.
Now, we know the common chord of two conics is given by equation
Where,
So, both the equations of circle and parabola are given as
Now, from the equation (iii), we can get equation of common chord as
Now, as the above equation is quadratic in ‘x’, so, we can get values of ‘x’ using the quadratic formula to get roots of any quadratic equation
Hence, we get values of ‘x’ from equation (iv) and (v) as
So, we can get two values f ‘x’ as
Now, from the diagram, we can observe the common chord of the curves will lie in positive x-direction only so, we can ignore
So,
So, the point T, (where the common chord is meeting at x-axis) is given as
Now, we know mid-point of any two points
So, mid-point of focus F(a, o) and vertex 0(o, o) can be given as
Hence, the point
So, the common chord is bisecting the focus and vertex.
Hence, the given statement is proved.
Note: One may solve the given equations of circle and parabola to get the equation of common chord. As
Fundamental concepts of parabola and ellipse should be very clear to solve these kinds of questions.
Another approach for the question would be to suppose the equation of common chord as
y = mx + c and try to solve it with the circle and parabola both. And hence, get the intersection points to calculate the equation of the common chord passing through both of the curves. But it will be a longer approach than given in solution. So, always try to solve these questions by visualizing the calculation part as well.
Complete step-by-step answer:
Let us suppose the equation of parabola be
So, diagram of parabola is given as

Where (a, o) is the focus of the parabola.
Now, a circle is drawn with center at the vertex i.e. at (o, o) and diameter of
Now, we know the standard equation of the circle is given by equation
Where,
So, equation of the circle with (0, 0) as centre and radius as
Now, we have to prove that the common chord of the circle and parabola bisects the distance between vertex and focus.
So, diagram with the help of above information is given as:

Where line L is the common chord of parabola and circle, as given in the diagram and F(a, 0) is the focus of the parabola.
Now, we know the common chord of two conics is given by equation
Where,
So, both the equations of circle and parabola are given as
Now, from the equation (iii), we can get equation of common chord as
Now, as the above equation is quadratic in ‘x’, so, we can get values of ‘x’ using the quadratic formula to get roots of any quadratic equation
Hence, we get values of ‘x’ from equation (iv) and (v) as
So, we can get two values f ‘x’ as
Now, from the diagram, we can observe the common chord of the curves will lie in positive x-direction only so, we can ignore
So,
So, the point T, (where the common chord is meeting at x-axis) is given as
Now, we know mid-point of any two points
So, mid-point of focus F(a, o) and vertex 0(o, o) can be given as
Hence, the point
So, the common chord is bisecting the focus and vertex.
Hence, the given statement is proved.
Note: One may solve the given equations of circle and parabola to get the equation of common chord. As
Fundamental concepts of parabola and ellipse should be very clear to solve these kinds of questions.
Another approach for the question would be to suppose the equation of common chord as
y = mx + c and try to solve it with the circle and parabola both. And hence, get the intersection points to calculate the equation of the common chord passing through both of the curves. But it will be a longer approach than given in solution. So, always try to solve these questions by visualizing the calculation part as well.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




