
A circle is inscribed in a regular hexagon of side
Answer
443.4k+ views
Hint:To solve this problem we should know about concept of circumscribed as well as basic trigonometry
Circumscribed: Circumscribed of a polygon is a circle that passes through all the polygon. The center of this circle is called the circumcenter and its radius is called the circumradius.
A trigonometric property,
Complete step by step answer:
As given in question we will draw diagram,
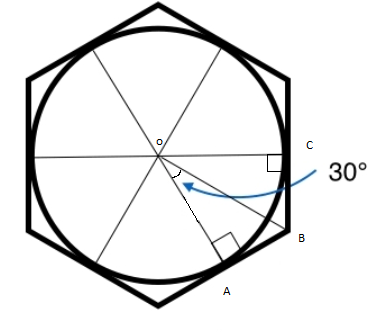
It is obvious from figure that
Let, there are two triangle AOB and COB,
In,
And
So, by RHS criteria both triangles are congruent.
Hence,
And
So,
In,
As, length of the side of the hexagon is
So,
(As
So circumference of the inscribed circle
Note: properties of regular hexagon:
It has six sides and six angles and the measurements of all angles are equal.
The total number of diagonals in a regular hexagon is nine.
The sum of all interior angles is equal to
Circumscribed: Circumscribed of a polygon is a circle that passes through all the polygon. The center of this circle is called the circumcenter and its radius is called the circumradius.
A trigonometric property,
Complete step by step answer:
As given in question we will draw diagram,

It is obvious from figure that
Let, there are two triangle AOB and COB,
In,
And
So, by RHS criteria both triangles are congruent.
Hence,
And
So,
In,
As, length of the side of the hexagon is
So,
(As
So circumference of the inscribed circle
Note: properties of regular hexagon:
It has six sides and six angles and the measurements of all angles are equal.
The total number of diagonals in a regular hexagon is nine.
The sum of all interior angles is equal to
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




