Question

A circle is inscribed in a square and then a smaller square is inscribed in the circle. The ratio of the area of the smaller square to that of the larger square is
(A) $ 1:4 $
(B) $ \sqrt{2}:2 $
(C) $ 1:2 $
(D) $ 1:\sqrt{2} $
(E) $ 2:3 $

Answer 1

Hint: For answering this question we should consider the two squares from the given information we have a circle is inscribed in a square and then a smaller square is inscribed in the circle. As the circle is inscribed inside the larger square we can say that the length of the side of the larger square will be equal to the diameter of the circle that is $ AD=2r $ here $ r $ is the assumed radius of the circle.

Complete step by step answer:
Now by considering the information given in the question we have a circle is inscribed in a square and then a smaller square is inscribed in the circle.

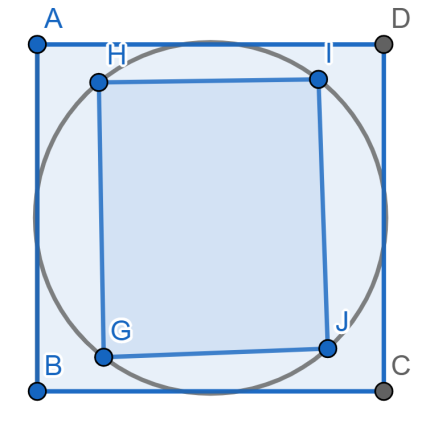
As the circle is inscribed inside the larger square we can say that the length of the side of the larger square will be equal to the diameter of the circle that is $ AD=2r $ here $ r $ is the assumed radius of the circle.
Hence we can say that the area of the larger square will be $ {{\left( 2r \right)}^{2}}=4{{r}^{2}} $ .
Let us consider the square $ GHIJ $ if we observe it carefully. The diagonals of this square acts similarly to the diameters of the circle.
Now from the Pythagoras theorem, we can say,
 $ H{{G}^{2}}+H{{I}^{2}}=G{{I}^{2}} $
By substituting the length of the diameter in the place of the length of the diagonal since they both are the same. We will have
 $ H{{G}^{2}}+H{{I}^{2}}={{\left( 2r \right)}^{2}} $
As it is a square $ HG=HI $ .
Let us assume $ HG=x $ then we will have
  $ \begin{align}
  & {{x}^{2}}+{{x}^{2}}=4{{r}^{2}} \\
 & \Rightarrow 2{{x}^{2}}=4{{r}^{2}} \\
 & \Rightarrow {{x}^{2}}=2{{r}^{2}} \\
 & \Rightarrow x=\sqrt{2}r \\
\end{align} $
Therefore we can say that the length of the side of the smaller square is $ \sqrt{2}r $ . Hence we can conclude that the area of the smaller square will be $ {{\left( \sqrt{2}r \right)}^{2}}=2{{r}^{2}} $
We have been given that to find the ratio of area of the smaller square to that of the area of the larger square, that is equal to $ \dfrac{area\text{ of smaller square}}{\text{area of larger square}} $
By substituting the values we have we will get
 $ \dfrac{2{{r}^{2}}}{4{{r}^{2}}}=\dfrac{1}{2} $
Therefore the ratio of the area of the smaller square to that of the larger square is $ 1:2 $ .
So, option C is the correct option.

Note:
 While answering questions of this type we should be sure with our calculations and Pythagoras theorem. We know that the Pythagoras theorem is given as “In a right angle triangle, the square of the length of the largest side will be equal to the sum of the squares of the other two sides”. And if we clearly observe a square has 2 triangles inside it so there we will have the square of the length of the diagonal equal to twice the sum of the square of the length of the side of the square.