Question

A circle is inscribed in an equilateral triangle ABC with side 12 cm, touching its sides. Find the radius of the inscribed circle and the area of the shaded part.

Answer 1

Hint: Here we go through by joining the perpendicular lines from the center of the circle to the touching edge of the triangle and make three parts of the triangle and find their area and equate it with the total area to find out the radius of the circle.

Complete step-by-step answer:
Here in the question it is given that a circle is inscribed in an equilateral triangle ABC with side 12 cm.
Now first of all joined the line from center to the touching edge of the triangle.

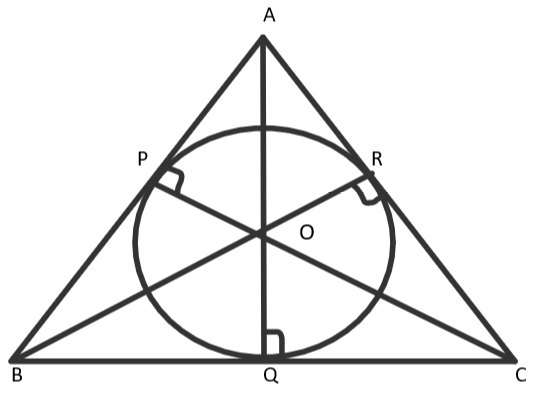
Here in the question ABC is an equilateral triangle it means AB=BC=AC=12cm.
Now after joining the lines from the center of the circle we can say that OP=OR=OQ=r, where ‘r’ is the radius of the circle.
We have O as the incenter and OP, OQ and OR are equal.
We can say that by figuring the sum of the triangles AOB, BOC and AOC is equal to the area of triangle ABC.
In $\vartriangle AOB$, $OP \bot AB$ because we know that the line joining from the center of the circle to the tangent of the circle where it touches the circle is perpendicular to it.
Similarly in $\vartriangle COB$, $OQ \bot BC$ and in$\vartriangle AOC$, $OR \bot AC$.
So we can write it as,
\[ \Rightarrow \;\;ar(\vartriangle ABC) = ar(\vartriangle OAB) + ar(\vartriangle OBC) + ar(\vartriangle OCA)\]
And we know the formula of the area of the equilateral triangle and the area of the triangle whose height and base are given.
$ \Rightarrow \dfrac{{\sqrt 3 }}{4} \times {(side)^2} = \left( {\dfrac{1}{2} \times OP \times AB} \right) \times \left( {\dfrac{1}{2} \times OQ \times BC} \right) \times \left( {\dfrac{1}{2} \times OR \times AC} \right)$
Now put the given values in the formula we get,
$
   \Rightarrow \dfrac{{\sqrt 3 }}{4} \times {(12)^2} = \left( {\dfrac{1}{2} \times r \times 12} \right) \times \left( {\dfrac{1}{2} \times r \times 12} \right) \times \left( {\dfrac{1}{2} \times r \times 12} \right) \\
   \Rightarrow \dfrac{{\sqrt 3 }}{4} \times {(12)^2} = 3\left( {\dfrac{1}{2} \times r \times 12} \right) \\
   \Rightarrow r = \dfrac{{36\sqrt 3 }}{{18}} \\
  \therefore r = 2\sqrt 3 cm \\
 $
Area of the shaded region = Area of $\vartriangle ABC$ - Area of circle.
$ \Rightarrow $ Area of the shaded region=$\dfrac{{\sqrt 3 }}{4} \times {(12)^2} - \dfrac{{22}}{7} \times {(2\sqrt 3 )^2} = (62.35 - 37.71)c{m^2} = 24.64c{m^2}$.

Note: Whenever we face such a type of question the key concept for solving the question is to first draw the diagram in that way that is useful in our calculation here in this question we have to find the radius of the circle so we draw the radius touching the side of the triangle and then by applying the formula of area of triangle we will easily solve our question.