Question

A circle is touching the side BC of the triangle ABC at P and touching AB and AC produced at Q and R, respectively. Prove that $AQ=AR=\dfrac{1}{2}\left( \text{Perimeter of }\Delta ABC \right)$ .

Answer 1

Hint: Start by drawing a neat diagram followed by using the theorem that the tangents to a circle from a given point outside the circle are equal. Remember that the perimeter of the triangle is equal to the sum of the three sides of the triangle.

Complete step-by-step solution -
First, we will draw a neat diagram of the situation given in the question.

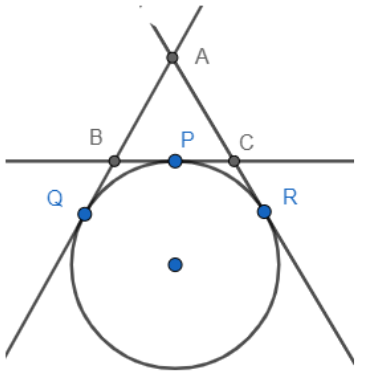
We know that the tangents drawn from a given point to the circle are equal. And it is clear from the figure that AQ and AR are tangents drawn from point A, BP, and BQ are tangents from point B, and CP and CR are tangents from point C.
$\therefore AQ=AR.............(i)$
$\therefore BP=BQ...........(ii)$
$\therefore CR=CP...........(iii)$
Now we know that the perimeter of the triangle is equal to the sum of the three sides of the triangle.
$\therefore \text{Perimeter of }\Delta ABC=AB+BC+CA$
Using the diagram, we can deduce that BC=BP+PC and CA=AR-CR. So, our equation becomes:
$\text{Perimeter of }\Delta ABC=AB+BP+PC+AR-CR$
Now, If we substitute the required values from equation (i), (ii) and (iii), we get
$\text{Perimeter of }\Delta ABC=AB+BQ+PC+AQ-PC$
Again, using the figure, we can say that AB+BQ=AQ.
$\text{Perimeter of }\Delta ABC=AQ+AQ$
$\Rightarrow \text{Perimeter of }\Delta ABC=2AQ$
$\Rightarrow \dfrac{1}{2}\times \text{Perimeter of }\Delta ABC=AQ$
So, using equation (i) and the above result, we can say that we have proved $AQ=AR=\dfrac{1}{2}\left( \text{Perimeter of }\Delta ABC \right)$ .

Note: The circle shown in the diagram of the question is also called the excircle and the center of the excircle is the point of intersection of the interior angle bisector of the opposite angle and the exterior angle bisectors of the other two angles of the triangle.