
A circular lamina of radius a and centre O has a mass per unit area of
Answer
516.6k+ views
Hint: In order to find the moment of inertia of the given shape, we need the mass of the lamina to find the moment of inertia, so we will consider mass of a small element and in order to find the mass of the lamina we will integrate the mass for the element. Further we will use the formula of moment of inertia and finally compare with the given value.
Formula used-
Complete Step-by-Step solution:
Let's consider the small element of lamina at x distance from the centre of thickness dx as shown below.
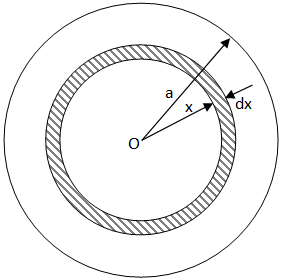
The area of the element is
The mass per unit area is given as
The mass of lamina is,
The moment of inertia of lamina about an axis through O and perpendicular to the lamina is,
Solve further,
As we know that:
Let us substitute the value of k in the equation of moment of inertia.
Thus, the required moment of inertia is
Hence, the value of x is 2.
Note- The moment of inertia of a solid body, otherwise known as the mass moment of inertia, angular momentum, or rotational inertia, is a quantity that specifies the torque necessary for a desired angular acceleration along a rotational axis; analogous to how gravity decides the force required for a desired acceleration. Students must remember the formula for moment of inertia for some common shapes and the general formula to find the moment of inertia.
Formula used-
Complete Step-by-Step solution:
Let's consider the small element of lamina at x distance from the centre of thickness dx as shown below.

The area of the element is
The mass per unit area is given as
The mass of lamina is,
The moment of inertia of lamina about an axis through O and perpendicular to the lamina is,
Solve further,
As we know that:
Let us substitute the value of k in the equation of moment of inertia.
Thus, the required moment of inertia is
Hence, the value of x is 2.
Note- The moment of inertia of a solid body, otherwise known as the mass moment of inertia, angular momentum, or rotational inertia, is a quantity that specifies the torque necessary for a desired angular acceleration along a rotational axis; analogous to how gravity decides the force required for a desired acceleration. Students must remember the formula for moment of inertia for some common shapes and the general formula to find the moment of inertia.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




