
A compound AB has simple cubic structure and has molecular mass 99. Its density is 3.4
Answer
481.2k+ views
Hint: The unit cell of a crystal is the smallest unit of the crystal lattice, a representation of three dimensional pattern of the entire crystal. As per assumptions, it is considered that in a crystal the same unit cell is repeated over and over again. The unit cell has opposite and parallel faces. The edge of a unit cell is connected to equivalent points. The unit cells are categorised into 7 categories, based on internal angles and edge lengths of the unit cell. The cubic lattice is one of the categories of unit cells.
Formula Used: The edge of unit cell can be determined by using following formula:
Here is the length of the edge of the unit cell. The letter Z represents the number of molecules per unit cell and M is the molar mass of the molecule. The density of the compound is e and
Complete step-by-step answer:
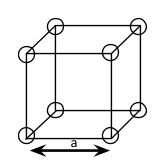
As given, the compound AB has simple cubic structure. The simple cubic unit cell has the lattice points present only at its corners. The simple cubic lattice unit cells are the smallest repetitive unit. The lattice point present at the corners are useful for ions, metals or molecules to have a crystalline structure. In simple cubic lattice, each unit cell is identical due to the fact that edges of each unit cell are equidistant. The unit cell in simple cubic lattice will have only one atom.
The given diagram shows a simple cubic lattice with lattice point only at its corners.
Step 1: First we need to obtain a formula for calculating the length of edge of the unit cell.
Density of unit cell
We know that,
But,
Here, M is the molar mass and
Also,
From formula (1) to (4), we know that
If we rearrange the formula (5), we get
Step 2: We have got the formula for finding out the edge of the unit cell of the simple cubic structure of compound AB.
For a simple cubic structure,
The Avogadro’s number,
The molar mass of compound AB,
The density of unit cell,
Let us substitute the respective values in formula 6 to obtain the value of
We have to take the cubic root of the above value to obtain the value of a.
Hence, the edge of the unit cell of cubic structure of compound AB is
Note:
-The simple cubic unit cell is a primitive cell that means the unit cell will have only one lattice point. -The number of Z that is the number of atoms present in a unit cell is 1. Hence, we have taken the value of Z as 1.
-The symbol, S
-Also, remember that the formula for density of a unit cell will provide us with the value of
Formula Used: The edge of unit cell can be determined by using following formula:
Here is the length of the edge of the unit cell. The letter Z represents the number of molecules per unit cell and M is the molar mass of the molecule. The density of the compound is e and
Complete step-by-step answer:
As given, the compound AB has simple cubic structure. The simple cubic unit cell has the lattice points present only at its corners. The simple cubic lattice unit cells are the smallest repetitive unit. The lattice point present at the corners are useful for ions, metals or molecules to have a crystalline structure. In simple cubic lattice, each unit cell is identical due to the fact that edges of each unit cell are equidistant. The unit cell in simple cubic lattice will have only one atom.
The given diagram shows a simple cubic lattice with lattice point only at its corners.
Step 1: First we need to obtain a formula for calculating the length of edge of the unit cell.

Density of unit cell
We know that,
But,
Here, M is the molar mass and
Also,
From formula (1) to (4), we know that
If we rearrange the formula (5), we get
Step 2: We have got the formula for finding out the edge of the unit cell of the simple cubic structure of compound AB.
For a simple cubic structure,
The Avogadro’s number,
The molar mass of compound AB,
The density of unit cell,
Let us substitute the respective values in formula 6 to obtain the value of
We have to take the cubic root of the above value to obtain the value of a.
Hence, the edge of the unit cell of cubic structure of compound AB is
Note:
-The simple cubic unit cell is a primitive cell that means the unit cell will have only one lattice point. -The number of Z that is the number of atoms present in a unit cell is 1. Hence, we have taken the value of Z as 1.
-The symbol, S
-Also, remember that the formula for density of a unit cell will provide us with the value of
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




