
A corn cob, shaped like a cone, has the radius of its broadest end as 1.4 cm and length (height) as 12 cm of the surface of the cob carries an average of four grains, find how many grains approximately you would find on the entire cob.
A) 211
B) 212
C) 213
D) 214
Answer
490.8k+ views
Hint:
It is given that r = 1.4 cm and h = 12 cm.
Now, using the formula
Thus, find the area of the cone by
Finally,
Complete step by step solution:
Here, it is given that the radius of its broadest end is 1.4 cm and length (height) as 12 cm of the surface of the cob.
Thus, r = 1.4 cm and
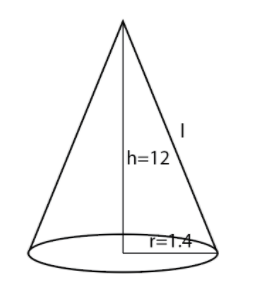
So, we get l using the formula
The C.S.A. of the cone is given by
Thus, the area of the cone cob is
It is also given that, number of corn grains in the
Thus, the number of corn grains carried in
So, option (C) is correct.
Note:
Here, the number 53.15 is rounded to 53.2 because the second digit after the decimal is 5, and if the digit after decimal is greater than or equal to 5 we add +1 to the previous digit i.e. 1 after the decimal.
Similarly, 212.8 is rounded off to 213, because the digit after decimal is greater than 5 i.e. 8, so we add +1 to 212 = 213.
It is given that r = 1.4 cm and h = 12 cm.
Now, using the formula
Thus, find the area of the cone by
Finally,
Complete step by step solution:
Here, it is given that the radius of its broadest end is 1.4 cm and length (height) as 12 cm of the surface of the cob.
Thus, r = 1.4 cm and

So, we get l using the formula
The C.S.A. of the cone is given by
Thus, the area of the cone cob is
It is also given that, number of corn grains in the
Thus, the number of corn grains carried in
So, option (C) is correct.
Note:
Here, the number 53.15 is rounded to 53.2 because the second digit after the decimal is 5, and if the digit after decimal is greater than or equal to 5 we add +1 to the previous digit i.e. 1 after the decimal.
Similarly, 212.8 is rounded off to 213, because the digit after decimal is greater than 5 i.e. 8, so we add +1 to 212 = 213.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Discuss the main reasons for poverty in India

What is the past participle of wear Is it worn or class 10 english CBSE

What is the full form of POSCO class 10 social science CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE




