
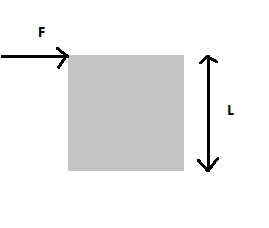
A cubical block of side L rests on a rough horizontal surface with the coefficient of friction
A. Infinitesimal
B.
C.
D.

Answer
485.4k+ views
Hint: In this question, we need to determine the minimum force required to topple the block such that the cubical block of side L rests on a rough horizontal surface with the coefficient of friction
Complete step by step answer:
Given the mass of block and is the side length of the block
For toppling, the normal reaction of the block comes to the extreme corner of the block touching the ground. Any further shift of normal reaction will result in the toppling. This is the limiting condition
Now if any force passes through the point of rotation it won’t produce any torque.
Thus here the normal reaction and the frictional force don’t produce any torque.
Now the torque produced by weight is anticlockwise while the torque by the applied force will be clockwise.
Since we want the minimum condition, we equate both the torques and we get that
So, the correct answer is “Option C”.
Note:
Remember that if the force passes through the point of rotation it won’t produce any torque. Torques should be balanced carefully. One should know the directions in which the torque is produced. Also remember that frictional force and normal reaction doesn’t produce any torque. So do not include them.
Complete step by step answer:
Given the mass of block and is the side length of the block
For toppling, the normal reaction of the block comes to the extreme corner of the block touching the ground. Any further shift of normal reaction will result in the toppling. This is the limiting condition
Now if any force passes through the point of rotation it won’t produce any torque.
Thus here the normal reaction and the frictional force don’t produce any torque.
Now the torque produced by weight is anticlockwise while the torque by the applied force will be clockwise.
Since we want the minimum condition, we equate both the torques and we get that
So, the correct answer is “Option C”.
Note:
Remember that if the force passes through the point of rotation it won’t produce any torque. Torques should be balanced carefully. One should know the directions in which the torque is produced. Also remember that frictional force and normal reaction doesn’t produce any torque. So do not include them.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




