
A cubical block of wood of side of 10 cm floats at the interface between oil and water as shown in figure with its lower face 2cm below the interface. The density of oil is
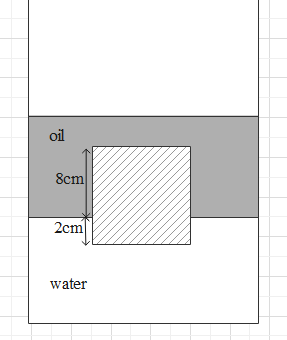
A. 600g
B. 680g
C. 800g
D. 200g

Answer
435.6k+ views
Hint: Since the block is floating, the net force on the block is zero. Therefore, equate the sum of buoyant forces exerted on the block by oil and water to the gravitational force on the body. Use
Formula used:
Complete step by step answer:
To solve this problem we need the concept of buoyancy.
When a body of mass m is submerged in a liquid, the liquid pushes the body upwards. This means that other than the gravitational force acting on the body, there is one more force exerted by the liquid. This force is called the force of buoyancy or buoyant force. The direction of this force is always upwards and opposite to the direction of the gravitational force.
The value of the buoyant force is given as
In this formula
We know that gravitational force is
In this case, both the liquids (water and oil) will exert upwards forces on the cubical block. And the gravity will exert a downward force.
Let the buoyant forces exerted by the oil and water be
The free diagram of the block will be:
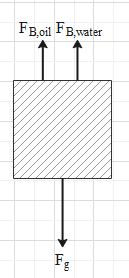
It is given that the block is floating. This means that the net force on the block is zero.
Therefore, the upward force balances the downward force.
Hence,
Let us calculate the value of
Let the density of oil be
Therefore,
It is given
And
Hence,
Let us calculate the value of
Let the density of oil be
Therefore,
And
Hence,
And
Substitute the values of
This means that the mass of the block is 680 grams.
Hence, the correct answer is option B.
Note:
Consider
When a block is submerged in a liquid, it will displace a volume of liquid, which is equal to
Now, by using
Substitute the value of
This means that the buoyant force is equal to the weight of the liquid displaced. Therefore, sometimes the buoyant force is referred to as the weight of the liquid displaced.
Formula used:
Complete step by step answer:
To solve this problem we need the concept of buoyancy.
When a body of mass m is submerged in a liquid, the liquid pushes the body upwards. This means that other than the gravitational force acting on the body, there is one more force exerted by the liquid. This force is called the force of buoyancy or buoyant force. The direction of this force is always upwards and opposite to the direction of the gravitational force.
The value of the buoyant force is given as
In this formula
We know that gravitational force is
In this case, both the liquids (water and oil) will exert upwards forces on the cubical block. And the gravity will exert a downward force.
Let the buoyant forces exerted by the oil and water be
The free diagram of the block will be:

It is given that the block is floating. This means that the net force on the block is zero.
Therefore, the upward force balances the downward force.
Hence,
Let us calculate the value of
Let the density of oil be
Therefore,
It is given
And
Hence,
Let us calculate the value of
Let the density of oil be
Therefore,
And
Hence,
And
Substitute the values of
This means that the mass of the block is 680 grams.
Hence, the correct answer is option B.
Note:
Consider
When a block is submerged in a liquid, it will displace a volume of liquid, which is equal to
Now, by using
Substitute the value of
This means that the buoyant force is equal to the weight of the liquid displaced. Therefore, sometimes the buoyant force is referred to as the weight of the liquid displaced.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




