
A cylindrical metallic rod in thermal contact with two reservoirs of heat at its two ends conducts an amount of heat
(A)
(B)
(C)
(D)
Answer
468.6k+ views
Hint To solve this question, we need to use the formula for the heat conducted across a conductor per unit time. We need to assume the dimensions of the rod and the temperature of the reservoir. Putting these in the formula, we will get the expression for
Formula used: The formula used to solve this question is given by
Complete step-by-step solution:
Let the length, the area of cross section, and the thermal conductivity of the metallic rod be
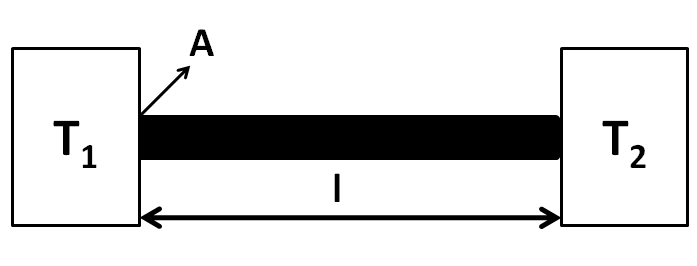
We know that the rate of heat conducted per unit time is given by
Substituting
Now, according to the question, the rod is melted and from that a new rod of half the radius is formed. We know that the area of cross section is related to the radius as
Putting
Putting (3) in the above equation, we get
Since the material of the rod is still the same, there is no change in the thermal conductivity. Also, there is no change in the length and the temperature of the reservoirs. Therefore, if
Dividing (5) by (2) we get
Putting (4) above, we get
Hence, the correct answer is option A.
Note: If we do not remember the formula for the heat conducted per unit time, then we can use the analogy between the electric current and the heat to derive the same. As we know that the resistance is given by
Formula used: The formula used to solve this question is given by
Complete step-by-step solution:
Let the length, the area of cross section, and the thermal conductivity of the metallic rod be

We know that the rate of heat conducted per unit time is given by
Substituting
Now, according to the question, the rod is melted and from that a new rod of half the radius is formed. We know that the area of cross section is related to the radius as
Putting
Putting (3) in the above equation, we get
Since the material of the rod is still the same, there is no change in the thermal conductivity. Also, there is no change in the length and the temperature of the reservoirs. Therefore, if
Dividing (5) by (2) we get
Putting (4) above, we get
Hence, the correct answer is option A.
Note: If we do not remember the formula for the heat conducted per unit time, then we can use the analogy between the electric current and the heat to derive the same. As we know that the resistance is given by
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




