
A cylindrical pillar is 50cm in diameter and 3.5m in height, find the cost of painting the curved surface of the pillar t the rate of Rs 12.50 per
Answer
508.8k+ views
Hint:- The approach for solving this problem is – we have to first find the curved surface area of the pillar in
Complete step by step solution:
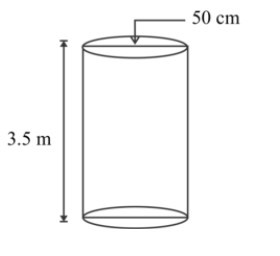
Given data,
Diameter = 50cm
Radius = 25cm
=0.25m
Height of the pillar
H = 3.5m
At first, we have to find the curved surface of the pillar.
We know that the curved surface area of a cylinder
The total cost
So, the total painting cost is Rs 68.75.
Note: We have to very careful about where the painting is applied, because if paint is applied on both the upper surface and lower surface then we have to find the total surface area for that care by this formula
Complete step by step solution:

Given data,
Diameter = 50cm
Radius = 25cm
=0.25m
Height of the pillar
H = 3.5m
At first, we have to find the curved surface of the pillar.
We know that the curved surface area of a cylinder
The total cost
So, the total painting cost is Rs 68.75.
Note: We have to very careful about where the painting is applied, because if paint is applied on both the upper surface and lower surface then we have to find the total surface area for that care by this formula
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




