Question

(a) Define (i) unit vector (ii) negative vectors
(b) An aeroplane takes off at an angle of $30{}^\circ $ to horizontal. If the component of its velocity along the horizontal is $250km/hr$, what is actual velocity. Find also the vertical component of velocity.

Answer 1

Hint: In the first part of the question, we are asked to define some of the basic terms related to vectors. In the second part we have a basic numerical problem. You could represent the vector and its components in a neat diagram and then find the actual velocity and vertical component of velocity accordingly.

Complete answer:
(a) Unit vector:
We know that any physical quantity that has both magnitude as well as direction is categorized under vector quantity. If the magnitude of this vector is unity that is, 1, then that vector is called a unit vector. The unit vectors are otherwise termed as direction vectors.
Negative vectors:
This is actually a relative term, that is, we could say negative vector with reference to another vector. Consider two vectors of equal magnitude but opposite in directions then we could call that pair negative vectors. Assigning a negative sign to a vector simply reverses its direction. So, vectors could termed negative with respect to another vector.
(b) We know that any vector can be resolved into its horizontal and vertical components. The situation given look like this,

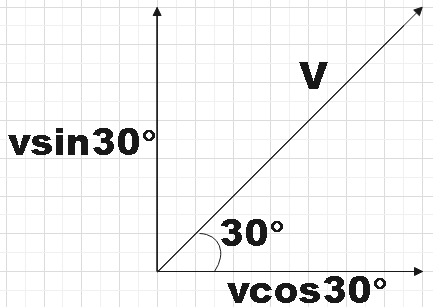
So, we are given the horizontal velocity to be 250km/hr, that is,
$v\cos 30{}^\circ =250km/hr$
$\Rightarrow v\left( \dfrac{\sqrt{3}}{2} \right)=250km/hr$
$\therefore v=\dfrac{250\times 2}{\sqrt{3}}=288.7km/hr$
Therefore, we found the actual velocity of the plane to be 288.7km/hr.
Vertical velocity would be,
$v\sin 30{}^\circ =288.7\times \dfrac{1}{2}$
$v\sin 30{}^\circ =144.35km/hr$
Hence, we found the vertical component of velocity to be 144.35km/hr.

Note:
Be it any other vector quantity the vertical and horizontal components would still be as taken as in the above solution. That is the cosine component of the vector would be the horizontal one and the sine component would be the vertical one. Also, one should be careful to check with respect to which component is the angle given.