Answer
430.2k+ views
Hint To solve this question, we need to use the principle of conservation of energy. Since the track is smooth, no frictional force will act on the disc. The only force which will act is the conservative gravitational force, so that the total mechanical energy of the disc will be conserved. So we need to find the total kinetic energy of the disc, which will be the sum of the translational and the kinetic energy of the disc. Then this energy has to be equated with the potential energy at the highest point to get the final value of the height.
Formula used: The formulae used to solve this question are
${K_T} = \dfrac{1}{2}m{v^2}$
${K_R} = \dfrac{1}{2}I{\omega ^2}$
$v = \omega R$
$U = mgh$
$I = \dfrac{1}{2}m{R^2}$
Complete step-by-step solution:
Let $m$ be the mass of the disc.
Since the disc is rolling as well as translating forward, its kinetic energy will be the sum of the translational and the rotational components. So the kinetic energy is given by
$K = {K_T} + {K_R}$
$ \Rightarrow K = \dfrac{1}{2}m{v^2} + \dfrac{1}{2}I{\omega ^2}$ ………………….(1)
It is given that the disc is rolling without sliding. Therefore, we have
$v = \omega R$
$ \Rightarrow \omega = \dfrac{v}{R}$ ……………………..(2)
Also, we know that the moment of inertia of a disc is given by
$I = \dfrac{1}{2}m{R^2}$..........................(3)
Substituting (2) and (3) in (1) we get
$K = \dfrac{1}{2}m{v^2} + \dfrac{1}{2}\left( {\dfrac{1}{2}m{R^2}} \right){\left( {\dfrac{v}{R}} \right)^2}$
$ \Rightarrow K = \dfrac{1}{2}m{v^2} + \dfrac{1}{4}m{R^2}\left( {\dfrac{{{v^2}}}{{{R^2}}}} \right)$
On simplifying, we get
$K = \dfrac{1}{2}m{v^2} + \dfrac{1}{4}m{v^2}$
$ \Rightarrow K = \dfrac{3}{4}m{v^2}$ ……………………….(4)
Let us take the potential energy at the horizontal level equal to zero. So the total energy of the disc at the horizontal level is equal to the kinetic energy, that is,
${E_1} = K$
$ \Rightarrow {E_1} = \dfrac{3}{4}m{v^2}$............................(5)
Now, let $h$ be the maximum height ascended by the disc. So the potential energy of the disc at the highest point is given by
$U = mgh$
The kinetic energy of the disc at the highest point will be equal to zero. So total mechanical energy of the disc at the highest point is equal to the potential energy, that is,
${E_2} = U$
$ \Rightarrow {E_2} = mgh$.............................(6)
Since no non-conservative force act on the disc, its mechanical energy is conserved, that is,
${E_1} = {E_2}$
Putting (5) and (6) we get
$\dfrac{3}{4}m{v^2} = mgh$
Cancelling $m$ from both sides
$\dfrac{3}{4}{v^2} = gh$
$ \Rightarrow h = \dfrac{{3{v^2}}}{{4g}}$
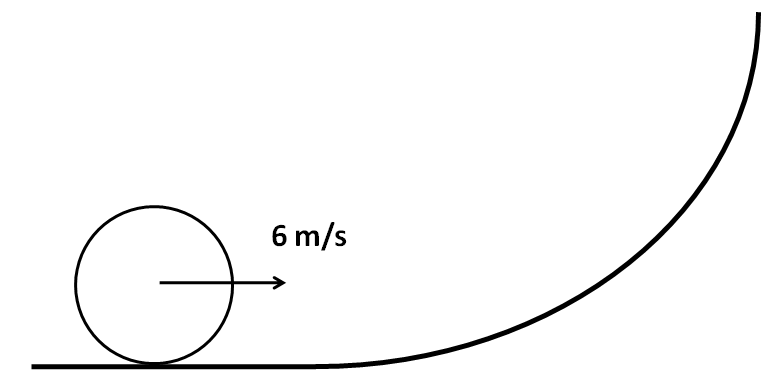
According to the question, $v = 6m{s^{ - 1}}$ and $g = 10m{s^{ - 2}}$. Putting these above, we finally get
$h = \dfrac{{3{{\left( 6 \right)}^2}}}{{40}}$
$ \Rightarrow h = 2.7m$
Hence, the correct answer is option C.
Note: Do not forget to calculate the rotational kinetic energy of the disc along with the translational kinetic energy. This is done since the disc is not only translating, but also rotating. We can also attempt this question using the work energy theorem. For that, we have to calculate the work done by the gravitational force, and equate it with the change in the kinetic energy of the disc to get the same equation as obtained in the above solution.
Formula used: The formulae used to solve this question are
${K_T} = \dfrac{1}{2}m{v^2}$
${K_R} = \dfrac{1}{2}I{\omega ^2}$
$v = \omega R$
$U = mgh$
$I = \dfrac{1}{2}m{R^2}$
Complete step-by-step solution:
Let $m$ be the mass of the disc.
Since the disc is rolling as well as translating forward, its kinetic energy will be the sum of the translational and the rotational components. So the kinetic energy is given by
$K = {K_T} + {K_R}$
$ \Rightarrow K = \dfrac{1}{2}m{v^2} + \dfrac{1}{2}I{\omega ^2}$ ………………….(1)
It is given that the disc is rolling without sliding. Therefore, we have
$v = \omega R$
$ \Rightarrow \omega = \dfrac{v}{R}$ ……………………..(2)
Also, we know that the moment of inertia of a disc is given by
$I = \dfrac{1}{2}m{R^2}$..........................(3)
Substituting (2) and (3) in (1) we get
$K = \dfrac{1}{2}m{v^2} + \dfrac{1}{2}\left( {\dfrac{1}{2}m{R^2}} \right){\left( {\dfrac{v}{R}} \right)^2}$
$ \Rightarrow K = \dfrac{1}{2}m{v^2} + \dfrac{1}{4}m{R^2}\left( {\dfrac{{{v^2}}}{{{R^2}}}} \right)$
On simplifying, we get
$K = \dfrac{1}{2}m{v^2} + \dfrac{1}{4}m{v^2}$
$ \Rightarrow K = \dfrac{3}{4}m{v^2}$ ……………………….(4)
Let us take the potential energy at the horizontal level equal to zero. So the total energy of the disc at the horizontal level is equal to the kinetic energy, that is,
${E_1} = K$
$ \Rightarrow {E_1} = \dfrac{3}{4}m{v^2}$............................(5)
Now, let $h$ be the maximum height ascended by the disc. So the potential energy of the disc at the highest point is given by
$U = mgh$
The kinetic energy of the disc at the highest point will be equal to zero. So total mechanical energy of the disc at the highest point is equal to the potential energy, that is,
${E_2} = U$
$ \Rightarrow {E_2} = mgh$.............................(6)
Since no non-conservative force act on the disc, its mechanical energy is conserved, that is,
${E_1} = {E_2}$
Putting (5) and (6) we get
$\dfrac{3}{4}m{v^2} = mgh$
Cancelling $m$ from both sides
$\dfrac{3}{4}{v^2} = gh$
$ \Rightarrow h = \dfrac{{3{v^2}}}{{4g}}$
According to the question, $v = 6m{s^{ - 1}}$ and $g = 10m{s^{ - 2}}$. Putting these above, we finally get
$h = \dfrac{{3{{\left( 6 \right)}^2}}}{{40}}$
$ \Rightarrow h = 2.7m$
Hence, the correct answer is option C.
Note: Do not forget to calculate the rotational kinetic energy of the disc along with the translational kinetic energy. This is done since the disc is not only translating, but also rotating. We can also attempt this question using the work energy theorem. For that, we have to calculate the work done by the gravitational force, and equate it with the change in the kinetic energy of the disc to get the same equation as obtained in the above solution.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

What is the meaning of celestial class 10 social science CBSE

What causes groundwater depletion How can it be re class 10 chemistry CBSE

Under which different types can the following changes class 10 physics CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Give 10 examples for herbs , shrubs , climbers , creepers