Question

(a) Draw a ray diagram showing the image formation by a compound microscope. Hence obtain expression for total magnification when the image is formed at infinity.
(b) Distinguish between myopia and hypermetropia. Show diagrammatically.

Answer 1

Hint: We need to know the basic criteria for drawing ray diagrams to symbolically represent the image formation and magnification by a compound microscope. The defects of the human eye can be rectified with proper lens combinations.

Complete step-by-step answer:
A compound microscope is combination of convex lenses which can produce an enlarged image of the object placed in front of the combination. We have to keep in mind that the image formed due to the first convex lens or the objective and the focal point of the second convex lens or the eyepiece should be coinciding at F as shown in the figure.

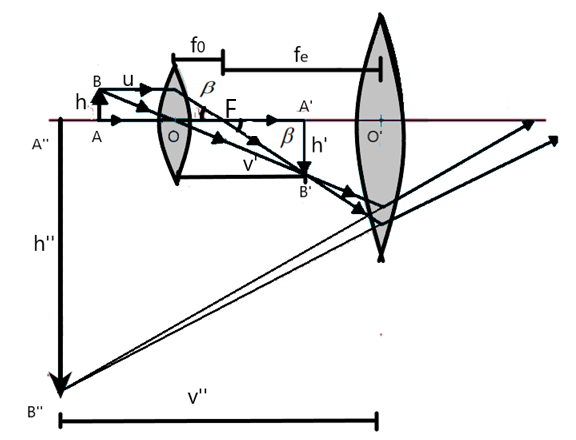
We can find the magnification of the final image as the product of the magnification caused by the objective and the eyepiece. The magnification of objective can be given as –
$$m_0={\displaystyle \frac{h^{\prime }}{h}}={\displaystyle \frac{A^{\prime }{B}^{\prime }}{AB}}$$
$$\Rightarrow m_0={\displaystyle \frac{L}{f_0}}$$
Where, L is the tube length defined as the length between the focal length of eyepiece and the focal length of the objective.
The magnification due to the eyepiece can be given as –
$$\Rightarrow m_e=1+{\displaystyle \frac{D}{f_e}}$$
Where, D is the least distance of distinct vision.
Now, the magnification of the compound microscope is given as –
$$\begin{array}{rl}& m=m_0{m}_e\\ & \Rightarrow m={\displaystyle \frac{L}{f_0}}(1+{\displaystyle \frac{D}{f_e}})\end{array}$$
For image at infinity the one factor can be removed.
The magnification becomes –
 $$\therefore m={\displaystyle \frac{L}{f_o}}{\displaystyle \frac{D}{f_e}}$$
(b) The two main defects of human eye are –
(i)Myopia – Also known as near-sightedness, the myopia is due to the formation of image before the retina due to the excess convergence of the eye lens. It can be corrected using a concave lens of proper focal length. The myopic condition is shown below.

(ii)Hypermetropia: This condition is due to the less convergence power of the eye ball resulting in an image formed beyond retina. We can use a convex lens of appropriate focal length to rectify this condition. The hypermetropic condition is shown below.

These are the solutions required for the problem.

Note: The microscopes are designed to obtain an image which is at infinity. This is to make the observer’s eyes more relaxed than straining to view the object. The improper use of lenses can cause defects to healthy eyes. The power should be appropriate for the user.