Question

When a eucalyptus tree is broken by strong wind, its top strikes the ground at an angle of ${{30}^{\circ }}$ to the ground and at a distance of 15m from the foot. What is the height of the tree?
A. $15\sqrt{3}m$
B. $10\sqrt{3}m$
C. $20m$
D. $10m$

Answer 1

Hint: For solving this question you should know about the general properties of a triangle and also know how to calculate the sides of a triangle if the angles of that triangle are given there. In this problem first we will make a diagram for this and then find the value of $\tan \theta $ at the given value of $\theta $ and thus we can find the height of the tree by using Pythagoras theorem.

Complete step by step answer:
According to our question, it is asked that when a eucalyptus tree is broken by strong wind, its top strikes the ground at an angle of ${{30}^{\circ }}$ to the ground and at a distance of 15m from the foot. What is the height of the tree? So, as we know that if any long trunk tree like eucalyptus and palm tree falls on the ground by breaking from the middle and if the trunk fully is not broken then it becomes a shape of a right angle triangle. And here the angle is ${{30}^{\circ }}$. So, if we make the diagram for it, then:

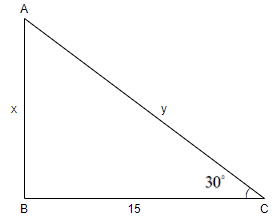
So, if we find the value of $\tan {{30}^{\circ }}$ here for getting the value of $y$, then:
From $\Delta ABC$,
$\begin{align}
  & \tan {{30}^{\circ }}=\dfrac{x}{15} \\
 & \Rightarrow \dfrac{1}{\sqrt{3}}=\dfrac{x}{15} \\
 & \Rightarrow x=5\sqrt{3} \\
\end{align}$
Now here we use the Pythagoras theorem:
$\begin{align}
  & {{y}^{2}}={{x}^{2}}+{{\left( 15 \right)}^{2}} \\
 & \Rightarrow {{y}^{2}}={{\left( 5\sqrt{3} \right)}^{2}}+{{\left( 15 \right)}^{2}} \\
 & \Rightarrow {{y}^{2}}=75+225 \\
 & \Rightarrow {{y}^{2}}=300 \\
\end{align}$
If we take the root, then:
$y=10\sqrt{3}$
So, the total height of the tree is,
$5\sqrt{3}+10\sqrt{3}=15\sqrt{3}$
So, the correct answer is “Option A”.

Note: While solving these types of questions you have to keep in mind that if there is any form of triangle, then find out if there is any triangle or not because if it is a triangle there then it is easy to solve that question otherwise it will be very tough.