Question

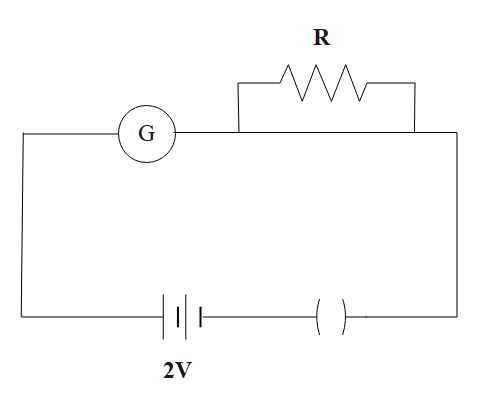
A galvanometer has a 50-division scale. The battery has no internal resistance. It is found that there is a deflection of 40 divisions when $R = 2400\Omega $. Deflection becomes 20 divisions when resistance taken from the resistance box is $4900\Omega $. Then we can conclude:

A. current sensitivity of galvanometer is $20\mu A/division$
B. resistance of galvanometer is $200\Omega $
C. resistance required on R.B for a deflection of 10 divisions is $9800\Omega $
D. full-scale deflection current is $2m.A$
E. Not solvable

Answer 1

Hint: We know that the current sensitivity of a moving coil galvanometer is defined as the current in a microampere needed to consume a deflection of one millimeter at a scale 1m away from the mirror.
Formula Used:
 Current Sensitivity $ = \dfrac{1}{{{\text{number of divisions}}}}$
${I_g} = \dfrac{V}{{R + G}}$

Complete step-by-step solution
Let full scale deflection of current = 1
As students know that, the voltmeter is a device, which is used to measure the potential difference between the two ends of a current-carrying conductor, and by connecting a high resistance in series with a galvanometer it can be converted into a voltmeter.
When a resistance ‘R’ is connected with the galvanometer in series, the current through the galvanometer is given as, ${I_g} = \dfrac{V}{{R + G}}$
In case 1, using the above relation when R = 2400 $\Omega $ and deflection of 40 divisions is present.
So, $\dfrac{{40}}{{50}}I = \dfrac{V}{{G + R}}$
$ \Rightarrow \dfrac{4}{5}I = \dfrac{2}{{G + 2400}}$ - (1)
Similarly, in case 2, when R = 4900 $\Omega $ and deflection of 20 divisions is present.
So, $\dfrac{{20}}{{50}}I = \dfrac{V}{{G + R}}$
$ \Rightarrow \dfrac{2}{5}I = \dfrac{V}{{G + 4900}}$ - (2)
From equation (1) and (2) we get,
$\dfrac{4}{2} = \dfrac{{G + 4900}}{{G + 2400}}$
$ \Rightarrow 2G + 4800 = G + 4900$
$ \Rightarrow G = 100\Omega $
Substituting G in equation (1) we will get,
$\dfrac{4}{5}I = \dfrac{2}{{100 + 2400}}$
$ \Rightarrow I = 1mA$
Current Sensitivity $ = \dfrac{1}{{{\text{number of divisions}}}}$
$ = \dfrac{1}{{50}}$ = 0.02mA/division$ = 20\mu A/division$
Resistance required for deflection of 10 divisions,
$\dfrac{{10}}{{50}}I = \dfrac{V}{{G + R}}$
$ \Rightarrow \dfrac{1}{5} \times 1 \times {10^{ - 3}} = \dfrac{2}{{100 + R}}$
$ \Rightarrow R = 9900\Omega $
Hence, option (A) is the correct answer.

Additional Information:
A galvanometer is a device used to measure the magnitude of the small electric current. The current and its strength is determined by the movement of a magnetic needle or that of a coil in a magnetic field. A moving coil galvanometer is a very sensitive device. The principle of the moving coil galvanometer is that when placed in an external magnetic field the current-carrying coil experiences magnetic torque.

Note: Current sensitivity of a moving coil galvanometer is defined as the deflection produced in the galvanometer when a unit current flows through it. If $d\theta $ is the change in the deflection produced by a small change in the current $dI$
So, the sensitivity of the galvanometer is given by
$S = \dfrac{{d\theta }}{{dI}}$
The current flowing through the moving coil galvanometer is given by
$I = \dfrac{k}{{nAB}}\theta $
where ‘$\theta $’ expresses the angle of deflection, ‘n’ is the number of turns, ‘A’ is the area, ‘B’ is the magnetic induction and ‘k’ is the couple per unit twist.