Question

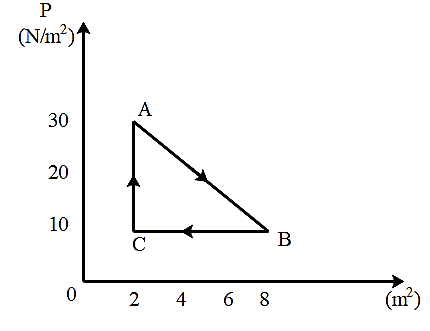
A gas undergoes the cyclic process shown in figure. The cycle is repeated 100 times per minute. The power generated is:

(A)$240W$
(B)$100W$
(C)$60W$
(D)$120W$

Answer 1

Hint: This question is given from chapter law of thermodynamics. Here, we will first see whether the work done by the gas is positive or negative depending on the direction of movement of gas. As we know that the work done by the gas is the area of the curve so we will calculate the area of the P-V curve. Now, we know that the expression for power is the ratio of the work done to the time taken. In this way, we will find the power generated.

Complete step by step answer:
As we all know that work done is equal to the area under the curve so,
$\left| w \right|\,=Area\,under\,the\,curve$
Now, as gas moves in clockwise direction so, work done will be positive i.e.
$w>0$
So, calculating work done –
$\begin {align}
  & w=\dfrac {1} {2}\times \left (30-10 \right)\left (8-2 \right) \\
 & \Rightarrow w=\dfrac {1} {2}\times 20\times 6 \\
 & \Rightarrow w=60J \\
\end{align}$
 As mentioned in the question that the cycle is repeated 100 times, so work done will be –
$\begin {align}
  & w=60\times 100 \\
 & \Rightarrow w=6000J \\
\end{align}$
Now, we will calculate power generated and for this we required work done and time taken,
$\begin {align}
  & Power=\frac {Work\, Done} {Time\, Taken} \\
 & \Rightarrow Power=\frac {6000} {60} \\
 & \therefore Power=100W \\
\end{align}$

So, the correct answer is “Option B”.

Note: We can solve this question by another method also i.e. by dividing the curve into 3 states and the calculating work done by each state and then, summing up the work done by all three states gives the total work done by the gas. Then, dividing the total work done by the time taken gives the value of power generated. After this by analyzing the direction of gas we can calculate whether the work done is positive or negative.