
A graph of the square of the velocity against the square of the acceleration of a given simple harmonic motion is:
A.
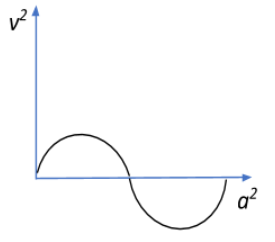
B.
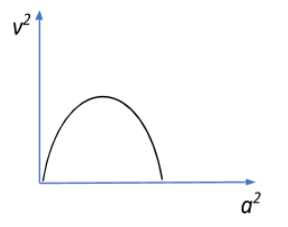
C.
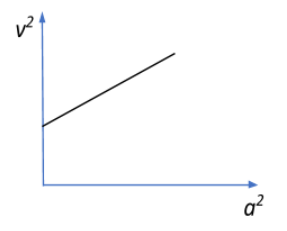
D.
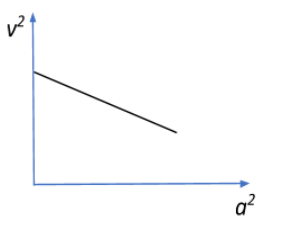




Answer
501.6k+ views
Hint: Begin with the equation for displacement of a particle performing SHM that is in terms of amplitude, phase and frequency. Take the time derivative of this equation to obtain the velocity equation and square it. Then take the time derivative of the velocity equation to obtain the acceleration and square this as well. Combine the two equations and strategically rearrange the terms in such a way that you are able to reproduce an equation for a plane curve that corresponds to the appropriate graph.
Formula Used:
Displacement of an object performing SHM:
Velocity of an object performing SHM:
Acceleration of an object performing SHM:
Complete answer:
A simple harmonic motion (SHM) is a type of periodic motion where the force acting on the oscillating object is directly proportional to the magnitude of the object’s displacement and is always directed towards the object’s equilibrium position. This is given as:
For any SHM, the displacement of its vibrating constituent at any instant of time t is given by:
The speed of the SHM oscillations at any instant of time t is given by taking the derivative of the above equation, i.e.,
The acceleration of the SHM oscillations is given by taking the derivative of the above equation:
Now, let us square the expressions we got for the velocity and acceleration of SHM oscillations:
Adding the above two equations, we get:
This equation represents an ellipse, whose general equation is:
Here,
Therefore, the graph closest to this approximation.
So hence the correct option, would be B.
Note:
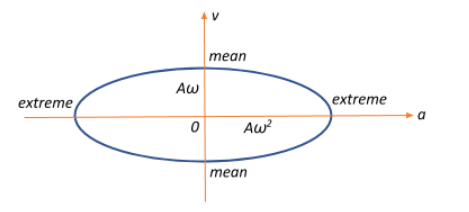
Remember that for a particle executing SHM, at mean position, the velocity of the particle is maximum and the acceleration of the particle is minimum, whereas at the extreme position, the velocity is minimum and the acceleration of the particle is maximum. This can be used to heuristically determine the shape of the graph between the velocity and acceleration, which ultimately is an ellipse:
Formula Used:
Displacement of an object performing SHM:
Velocity of an object performing SHM:
Acceleration of an object performing SHM:
Complete answer:
A simple harmonic motion (SHM) is a type of periodic motion where the force acting on the oscillating object is directly proportional to the magnitude of the object’s displacement and is always directed towards the object’s equilibrium position. This is given as:
For any SHM, the displacement of its vibrating constituent at any instant of time t is given by:
The speed of the SHM oscillations at any instant of time t is given by taking the derivative of the above equation, i.e.,
The acceleration of the SHM oscillations is given by taking the derivative of the above equation:
Now, let us square the expressions we got for the velocity and acceleration of SHM oscillations:
Adding the above two equations, we get:
This equation represents an ellipse, whose general equation is:
Here,
Therefore, the graph closest to this approximation.
So hence the correct option, would be B.
Note:
Remember that for a particle executing SHM, at mean position, the velocity of the particle is maximum and the acceleration of the particle is minimum, whereas at the extreme position, the velocity is minimum and the acceleration of the particle is maximum. This can be used to heuristically determine the shape of the graph between the velocity and acceleration, which ultimately is an ellipse:

Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




