
A group of 123 workers went to a canteen for coffee, ice-cream and tea. 42 workers took ice-cream, 36 took tea and 30 took coffee, 15 workers purchased ice-cream and tea, 10 ice-cream and coffee and 4 coffee and tea but not ice-cream, while 11 took ice-cream and tea but not coffee. Determine how many workers did not purchase anything?
Answer
491.4k+ views
Hint: We will proceed in this problem by making a venn diagram of the problem.
Let consider three sets i.e.,
Complete step-by-step answer:
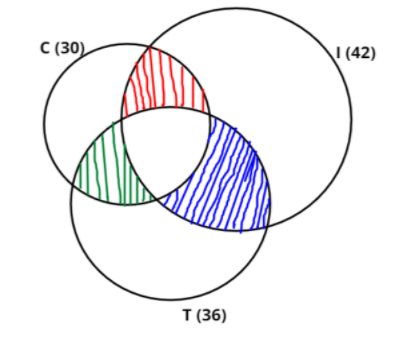
Number of workers purchasing ice-cream,
Number of workers purchasing tea,
Number of workers purchasing coffee,
Number of workers purchasing ice-cream and tea,
Number of workers purchasing ice-cream and coffee,
Number of workers purchasing only ice-cream and tea but not coffee (shown in the figure through blue coloured hatched lines) is given by
Number of workers purchasing only coffee and tea but not ice-cream (shown in the figure through green coloured hatched lines) is given by
As we know that for any three sets i.e.,
Now substituting all the values in equation (1), we get
Number of workers purchasing either ice-cream or tea or coffee is given by
Since, Number of workers who did not purchase anything is equal to the total number of workers minus the number of workers purchasing either ice-cream or tea or coffee.
Note: In these types of problems, a venn diagram is used to calculate all the unknowns. In this particular problem, we used the given data to determine the unknowns in equation (1).
Let consider three sets i.e.,
Complete step-by-step answer:

Number of workers purchasing ice-cream,
Number of workers purchasing tea,
Number of workers purchasing coffee,
Number of workers purchasing ice-cream and tea,
Number of workers purchasing ice-cream and coffee,
Number of workers purchasing only ice-cream and tea but not coffee (shown in the figure through blue coloured hatched lines) is given by
Number of workers purchasing only coffee and tea but not ice-cream (shown in the figure through green coloured hatched lines) is given by
As we know that for any three sets i.e.,
Now substituting all the values in equation (1), we get
Number of workers purchasing either ice-cream or tea or coffee is given by
Since, Number of workers who did not purchase anything is equal to the total number of workers minus the number of workers purchasing either ice-cream or tea or coffee.
Note: In these types of problems, a venn diagram is used to calculate all the unknowns. In this particular problem, we used the given data to determine the unknowns in equation (1).
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

The reason why India adopted the policy of nonalignment class 11 social science CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

The plastids which are coloured green and colourless class 11 biology CBSE




