
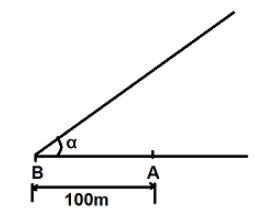
A gunman sitting in a shelter fires a bullet. The shelter is at an angle

Answer
456.6k+ views
Hint:In this question, it is given that the trajectory of the gun shot lies in the plane of the figure. However, we will consider the trajectory that touches the shelter from all the possible trajectories. By doing this, the angle at which the bullet should be fired to touch the shelter. And finally by the help of this angle, we will determine the maximum range of the gunshot.
Complete step by step answer:
We will first derive the formula for the maximum range of the gunshot and then put the value of the base given in the figure. Let the base be
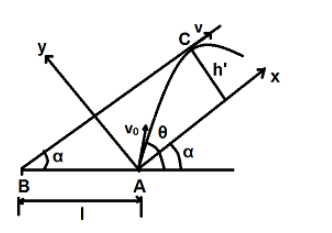
We can determine from the figure that the horizontal component of the initial velocity of the bullet in this system is
Point C at which the trajectory of the bullet touches the shelter gives us the maximum altitude of the shell above the horizontal which is given by:
We can thus write that
Now, the maximum range of the gunshot can be given by:
Now we will put
Hence,the maximum range of gunshot is
Note:In this question, we have used the coordination system for the trajectory when the bullet touches the shelter. We have considered the angle of projection in this trajectory only. Also, the maximum amplitude of the gunshot is considered in this trajectory. Therefore, we should not be confused between the original and the considered coordination system.
Complete step by step answer:
We will first derive the formula for the maximum range of the gunshot and then put the value of the base given in the figure. Let the base be

We can determine from the figure that the horizontal component of the initial velocity of the bullet in this system is
Point C at which the trajectory of the bullet touches the shelter gives us the maximum altitude of the shell above the horizontal which is given by:
We can thus write that
Now, the maximum range of the gunshot can be given by:
Now we will put
Hence,the maximum range of gunshot is
Note:In this question, we have used the coordination system for the trajectory when the bullet touches the shelter. We have considered the angle of projection in this trajectory only. Also, the maximum amplitude of the gunshot is considered in this trajectory. Therefore, we should not be confused between the original and the considered coordination system.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




