
A healthy young man standing at a distance of
Answer
491.4k+ views
Hint: You can begin by first calculating the amount of time the kid will take to reach the man at the bottom of the building. Keep in mind that the kid finally reaches the man’s arm height, so account for that in your estimation of the vertical distance that the kid travels. The time that you calculate for the kid is also the time that the man must take to cover the horizontal distance to the bottom of the building. Finding the velocity will now be pretty straightforward.
Formula used: Kinematic equation of motion:
Velocity
Complete step by step answer:
We are required to find the speed with which the man must run so that he reaches the bottom of the building just in time to save the kid.
We already know that he has to travel a distance of
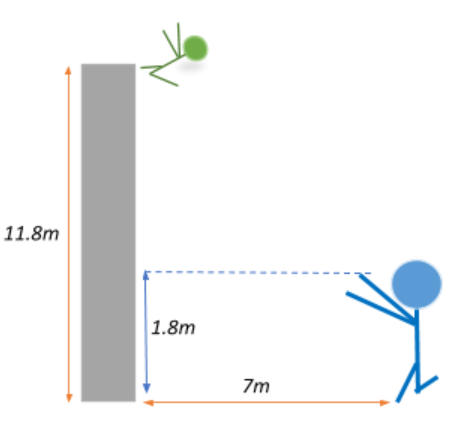
We can thus find the time the kid takes to slip all the way to the bottom of the building from a kinematic equation of motion:
Now, this will also be the maximum amount of time that the man can take to reach the bottom of the building. Therefore, for the man has to run with a velocity of:
Therefore, in order to catch the kid, the man has to run with a velocity of at least
Note: In the problem, do not overlook the fact that the kid is caught at the man’s arms height since it is only the man that will cover a horizontal distance to reach the bottom of the building while the kid is not necessarily caught at the bottom of the building. Note that the velocity that we obtain is the minimum velocity with which the man should run to be able to save the kid, and the time he takes is the maximum window that is available to save the kid, beyond which taking more time will not get him to the bottom of the building before the kid hits the ground.
Formula used: Kinematic equation of motion:
Velocity
Complete step by step answer:
We are required to find the speed with which the man must run so that he reaches the bottom of the building just in time to save the kid.
We already know that he has to travel a distance of

We can thus find the time the kid takes to slip all the way to the bottom of the building from a kinematic equation of motion:
Now, this will also be the maximum amount of time that the man can take to reach the bottom of the building. Therefore, for the man has to run with a velocity of:
Therefore, in order to catch the kid, the man has to run with a velocity of at least
Note: In the problem, do not overlook the fact that the kid is caught at the man’s arms height since it is only the man that will cover a horizontal distance to reach the bottom of the building while the kid is not necessarily caught at the bottom of the building. Note that the velocity that we obtain is the minimum velocity with which the man should run to be able to save the kid, and the time he takes is the maximum window that is available to save the kid, beyond which taking more time will not get him to the bottom of the building before the kid hits the ground.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




