
A hemispherical bowl is filled to the brim with a beverage. The contents of the bowl are transferred into a cylindrical vessel whose radius is 50% more than its height. If the diameter is the same for both the bowl and the cylinder, then the amount of the beverage that can be poured from the bowl into the cylindrical vessel is _________.
A.
B.
C. 100%
D. None of these
Answer
499.5k+ views
Hint: For solving this question we will need to find the volumes of the two vessels. The volume of a hemisphere is given by
Complete step by step answer:
Now, it is mentioned in the question that the hemispherical bowl is filled to the brim with the beverage.
Therefore, the volume of the hemispherical bowl is equal to the volume of the beverage.
Let the radius of the hemispherical bowl be ‘r’
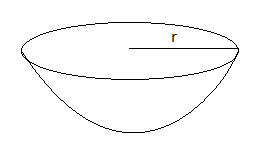
Thus, the volume of the beverage will be:
Now, we will find the volume of the cylindrical vessel.
It is given in the question that the diameter of both the hemispherical bowl and cylindrical vessel are equal. Hence, their radii are also equal.
Thus, the radius of the cylindrical vessel is ‘r’.
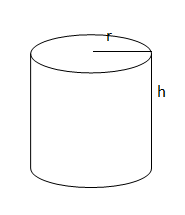
Now, it has been given to us that the radius of the cylindrical bowl is 50% more than the height of the vessel. Thus if the height of the vessel is ‘h’ then it is given as:
Now, we have both the radius and height of the cylinder in terms of ‘r’. Now we will calculate the volume of this vessel.
Volume of a cylindrical vessel is given as:
Now, we can see that the volume of the beverage is exactly equal to the volume of the cylindrical vessel. Thus, the beverage can be completely transferred from the hemispherical bowl to the cylindrical vessel and the cylindrical vessel will be filled up to the brim.
Thus, 100% of the beverage can be transferred from the bowl to the vessel.
So, the correct answer is “Option C”.
Note: Always take one reference variable in these types of questions like we have taken ‘r’ in this question. Comparison can only be done if the variables in both the quantities to be compared are the same and it is easy to compare one variable. Also, don’t put the value of
Complete step by step answer:
Now, it is mentioned in the question that the hemispherical bowl is filled to the brim with the beverage.
Therefore, the volume of the hemispherical bowl is equal to the volume of the beverage.
Let the radius of the hemispherical bowl be ‘r’

Thus, the volume of the beverage will be:
Now, we will find the volume of the cylindrical vessel.
It is given in the question that the diameter of both the hemispherical bowl and cylindrical vessel are equal. Hence, their radii are also equal.
Thus, the radius of the cylindrical vessel is ‘r’.

Now, it has been given to us that the radius of the cylindrical bowl is 50% more than the height of the vessel. Thus if the height of the vessel is ‘h’ then it is given as:
Now, we have both the radius and height of the cylinder in terms of ‘r’. Now we will calculate the volume of this vessel.
Volume of a cylindrical vessel is given as:
Now, we can see that the volume of the beverage is exactly equal to the volume of the cylindrical vessel. Thus, the beverage can be completely transferred from the hemispherical bowl to the cylindrical vessel and the cylindrical vessel will be filled up to the brim.
Thus, 100% of the beverage can be transferred from the bowl to the vessel.
So, the correct answer is “Option C”.
Note: Always take one reference variable in these types of questions like we have taken ‘r’ in this question. Comparison can only be done if the variables in both the quantities to be compared are the same and it is easy to compare one variable. Also, don’t put the value of
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




