
A hemispherical bowl of radius
Answer
485.4k+ views
Hint: The block and bowl are rotating with the same angular velocity. Due to the rotation a centripetal force acts on them. Given that, the radius makes an angle with the vertical axis. Since the block is not slipping on the surface of the block, forces acting on the block are balanced. Here, we can equate the horizontal and vertical components of forces acting on the block. Using the equations for both vertical and horizontal components of forces, we can find the angular velocity of the block.
Formula used:
Complete step by step answer:
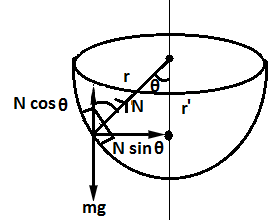
The centripetal force that the rotation exerts on the block,
Where,
Since, the block kept in the bowl rotates with the bowl, without slipping on its surface; the forces on the block should balance.
Here, since, the surface of the bowl is smooth; no frictional force is acting on the block. Therefore, the forces that act on the block are normal force
Horizontal and vertical components normal force are
Vertical forces on the block are equal. Then,
Where,
Similarly, the horizontal forces on the block are equal. Then,
Dividing the equation 2 by 1, we get,
Then,
The angular speed at which the ball is rotating is
Therefore, answer is option B.
Note:
A force that causes a uniform circular motion is known as centripetal force. The direction of a centripetal force is towards the center of curvature. Centripetal force is always perpendicular to the path and pointing to the center of curvature.
Formula used:
Complete step by step answer:

The centripetal force that the rotation exerts on the block,
Where,
Since, the block kept in the bowl rotates with the bowl, without slipping on its surface; the forces on the block should balance.
Here, since, the surface of the bowl is smooth; no frictional force is acting on the block. Therefore, the forces that act on the block are normal force
Horizontal and vertical components normal force are
Vertical forces on the block are equal. Then,
Where,
Similarly, the horizontal forces on the block are equal. Then,
Dividing the equation 2 by 1, we get,
Then,
The angular speed at which the ball is rotating is
Therefore, answer is option B.
Note:
A force that causes a uniform circular motion is known as centripetal force. The direction of a centripetal force is towards the center of curvature. Centripetal force is always perpendicular to the path and pointing to the center of curvature.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




