Question

A horizontal stretched string fixed at two ends, is vibrating in its fifth harmonic according the equation \[y(x,t)=0.01\sin [(62.8{{m}^{-1}}x)]\cos [(628{{s}^{-1}})t]\]. Assuming \[\pi =3.14\], the correct statement(s) is (are):
A. The number of nodes is 5
B. the length of the string is 0.25 m
C. The maximum displacement of the midpoint of the string, from its equilibrium position is 0.01 m
D. The fundamental frequency is 100 Hz.

Answer 1

Hint: In this question, we are given the equation of the wave. Now, we also know the general representation of the wave. So, comparing the two equations we will obtain the values of amplitude, angular frequency and the wave number. We have been given the statements in options and asked to choose the statements that are true for the given wave under the given conditions. We shall therefore evaluate each statement.

Complete answer:
The first statement says that the number of nodes in a wave vibrating in 5th harmonic is 5. Now, we know that A node is a point having zero amplitude. Therefore, from the figure below we can say that the number of nodes is 6.
                             

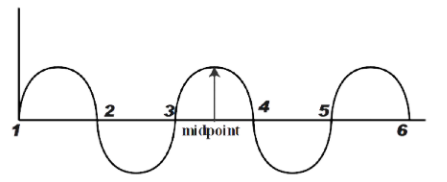
Therefore, the first statement is False.
The second statement says that the length of the string is 0.25m. Now from the above figure, we can say that
\[L=\dfrac{5\lambda }{2}\] …………………. (A)
Now, if we calculate \[\lambda \] we can find the total length of the string.
We know, the general equation of wave is,
\[y(x,t)=A\sin (kx\pm \omega t)\]
Comparing the general equation with given equation
\[y(x,t)=0.01\sin [(62.8{{m}^{-1}}x)]\cos [(628{{s}^{-1}})t]\]
We can say,
\[A=0.01;k=62.8;\omega =628\] …………………… (B)
We also know that
\[\lambda =\dfrac{2\pi }{k}\]
After substituting the value of k
We get,
\[\lambda =\dfrac{2\pi }{62.8}\]
On solving we get,
\[\lambda =0.1m\]
After substituting the value of \[\lambda \] in equation (A)
We get,
\[L=\dfrac{5\times 0.1}{2}\]
On solving
We get,
\[L=0.25m\]
Therefore, the length of the string is 0.25 m. So, the second statement is true.
Now, the third statement says that the maximum displacement of the midpoint of the string from its equilibrium position is 0.01. We know that the midpoint of the string is the point of maximum amplitude. Therefore, the maximum displacement of the midpoint of string from its equilibrium position will be equal to the amplitude.
From (B) we can say that amplitude is 0.01 m and therefore, the maximum displacement of the midpoint of string from its equilibrium position will also be 0.01 m.
Therefore, the third statement is true.
Now, the last statement says that the fundamental frequency is 100 Hz. We know fundamental frequency is the frequency of the first wave.
We know that the frequency of the wave for second (n=2) or higher harmonics (n=n) are the multiple of fundamental frequency (n=1)
 We know that frequency is given as
\[\omega =2\pi f\]
\[\therefore f=\dfrac{\omega }{2\pi }\]
  It is given that \[\omega =628\]for the fifth harmonic (n=5)
After substituting the value of \[\omega \], frequency of fifth harmonic is
\[{{f}_{5}}=\dfrac{628}{2\times 3.14}\]
Therefore,
\[{{f}_{5}}=100\]
Now, the fundamental frequency will be given by,
\[{{f}_{5}}=5{{f}_{1}}\]
Therefore,
\[{{f}_{1}}=\dfrac{100}{5}\]
Therefore,
\[{{f}_{1}}=20\]
Therefore, the last statement is false.

Therefore, the correct answer is option B, C.

Note:
The position for which the continuous displacement is zero is called a node. On the other hand, the position for maximum displacement is called a node. The distance between the two successive nodes is equal to half of the wavelength. The lowest frequency at which the medium freely oscillates is called the fundamental frequency.