
A hot liquid in a big room. The logarithm of the numerical value of the temperature difference between the liquid and the room is plotted against time. The plot will be very nearly
A) a straight line
B) a circular arc
C) a parabola
D) an ellipse
Answer
496.5k+ views
Hint: The hot liquid kept in a big room loses its temperature with time. The rate at which the liquid cools is directly proportional to the temperature difference between the liquid and the surroundings of the room. This is known as Newton’s law of cooling.
Complete step by step solution:
When a hot liquid is kept in a big room, the liquid will begin to lose its temperature with time and the surrounding walls of the room will gain the thermal energy of the liquid. Since the room is big, we can assume that the energy dissipated by the liquid will not change the temperature of the surroundings. According to Newton’s law of cooling, the rate of cooling of the hot liquid is directly proportional to the temperature difference between the liquid and the surroundings of the room. If T is the temperature of the hot liquid and
Where, k is a constant of proportionality. Now we bring the
Performing integration on both sides, we get
Where, C is the constant. From the above equation, we can say that
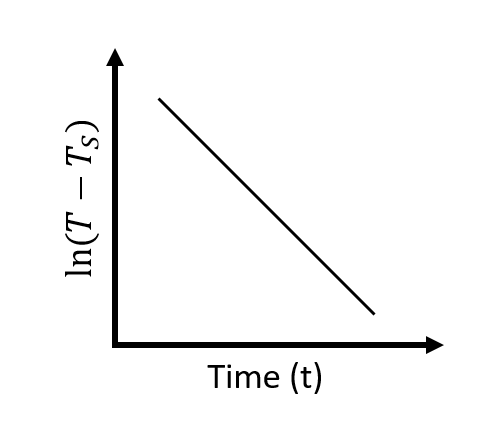
From the above relation, if a graph is plotted between
Note:
Newton’s law of cooling is only applicable to small temperature differences. So if the room would have been small the temperature difference would be large and hence, Newton’s law would not be applicable. In such cases, Stefan’s law is to be used.
Complete step by step solution:
When a hot liquid is kept in a big room, the liquid will begin to lose its temperature with time and the surrounding walls of the room will gain the thermal energy of the liquid. Since the room is big, we can assume that the energy dissipated by the liquid will not change the temperature of the surroundings. According to Newton’s law of cooling, the rate of cooling of the hot liquid is directly proportional to the temperature difference between the liquid and the surroundings of the room. If T is the temperature of the hot liquid and
Where, k is a constant of proportionality. Now we bring the
Performing integration on both sides, we get
Where, C is the constant. From the above equation, we can say that
From the above relation, if a graph is plotted between

Note:
Newton’s law of cooling is only applicable to small temperature differences. So if the room would have been small the temperature difference would be large and hence, Newton’s law would not be applicable. In such cases, Stefan’s law is to be used.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




