
A ladder of mass
(a). normal reaction of the wall on the ladder
(b). normal reaction of the ground on the ladder
(c). net force applied by the ground on the ladder

Answer
464.4k+ views
Hint: The ladder is in equilibrium against a wall, this means it is at rest or in uniform motion and no external forces act on it. We can apply Newton’s second law for forces acting in different directions and make equations. We can also apply equilibrium in its rotational motion and make equations for torque acting on it and solve the equations to calculate the value of forces.
Formulas used:
Complete answer:
The system of ladder is an isolated system, analyzing all the forces acting on it and resolving the forces into its components as follows
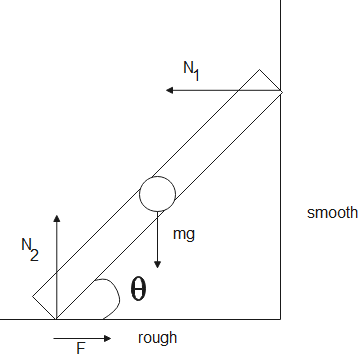
Since the ladder is on rest against the wall, the resultant of all forces acting on it will be 0.
Forces acting on the ladder in the x-direction
Forces acting in the y-direction
Now taking torque about point O, we have,
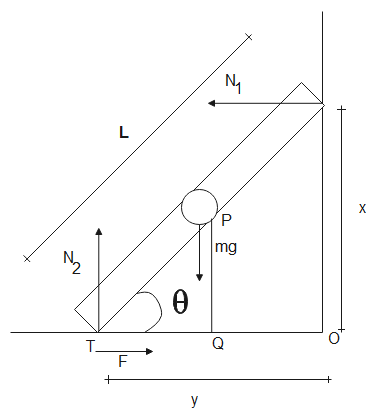
Since the ladder is in equilibrium, the net torque acting on it is 0.
Therefore, the torque is calculated as-
Here,
The axis is passing through point O
Distance of point of action of
From the triangle given in the above figure
Distance of point of action of
Distance of point of action of
Therefore, from eq (2), the torque acting on the ladder is-
Substituting values for ladder in the above equation, we get,
Therefore,
(a). The normal reaction of the wall on the ladder is
(b). The normal reaction of the ground on the ladder is
(c). the force applied by the ground on the ladder is
Note:
The negative sign indicates that the force is opposite to the assumed direction. The normal reaction is perpendicular to the surfaces and by Newton’s third law, it acts equal and opposite on both the surfaces. The frictional force resists the motion of a body; it is applied by rough surfaces. When an object is in equilibrium, no net force or torque acts on it.
Formulas used:
Complete answer:
The system of ladder is an isolated system, analyzing all the forces acting on it and resolving the forces into its components as follows

Since the ladder is on rest against the wall, the resultant of all forces acting on it will be 0.
Forces acting on the ladder in the x-direction
Forces acting in the y-direction
Now taking torque about point O, we have,

Since the ladder is in equilibrium, the net torque acting on it is 0.
Therefore, the torque is calculated as-
Here,
The axis is passing through point O
Distance of point of action of
From the triangle given in the above figure
Distance of point of action of
Distance of point of action of
Therefore, from eq (2), the torque acting on the ladder is-
Substituting values for ladder in the above equation, we get,
Therefore,
(a). The normal reaction of the wall on the ladder is
(b). The normal reaction of the ground on the ladder is
(c). the force applied by the ground on the ladder is
Note:
The negative sign indicates that the force is opposite to the assumed direction. The normal reaction is perpendicular to the surfaces and by Newton’s third law, it acts equal and opposite on both the surfaces. The frictional force resists the motion of a body; it is applied by rough surfaces. When an object is in equilibrium, no net force or torque acts on it.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




