
A legendary Dutch boy saved Holland by plugging a hole of diameter
If he pulled his finger out of the hole, during what time interval would the release water fill
Answer
423.9k+ views
Hint : In order to solve this equation, we will use the concept of Bernoulli theorem of fluid mechanics which states that “the sum of the pressure energy, Kinetic energy, and potential energy per unit mass of an incompressible, non-viscous fluid in a streamlined flow always remains a constant”.
Complete step-by-step solution:
Let us first draw the diagram. Let
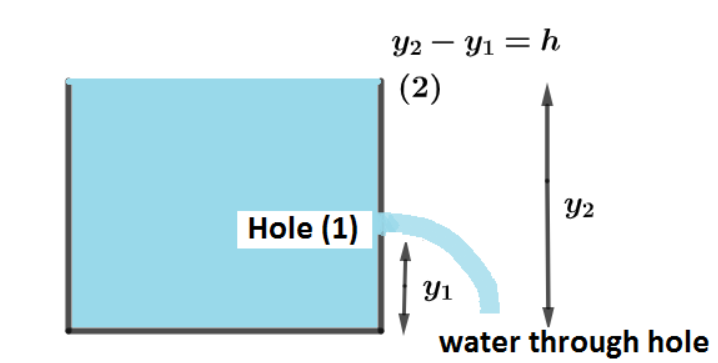
Now, we have
Let
Then by using Bernoulli theorem we can write as:
Where
Or
Put
On putting the values of
Now, Let
Now, as we know that volume of
So, in order to find time we can simple use
Or
Hence,
Note: It should be remembered that, the basic units of conversions are as
Complete step-by-step solution:
Let us first draw the diagram. Let

Now, we have
Let
Then by using Bernoulli theorem we can write as:
Where
Or
Put
On putting the values of
Now, Let
Now, as we know that volume of
So, in order to find time we can simple use
Or
Hence,
Note: It should be remembered that, the basic units of conversions are as
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




