Question

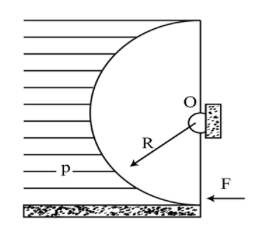
A light semi-cylindrical gate of radius R is pivoted at its midpoint O, of the diameter as shown in the figure holding liquid of density$\rho $. The force F is required to prevent the rotation of the gate is equal to
     

A.$2\pi {{\rm{R}}^3}\rho g$
B.$2\rho g{{\rm{R}}^3}l$
C. $\dfrac{{2{R^2}l\rho g}}{3}$
D. None of these

Answer 1

Hint:We know that fluid is a combination of liquids, gases and plasma. It has no definite shape; its pressure applies in all directions. Fluid pressure can also be amplified through the hydraulic mechanism and changes with the velocity of fluids.

Complete step by step answer:
According to the question, the volume of semi-cylinder with radius r is given by ${\rm{V}}\;{\rm{ = }}\;\pi {r^2}h$
Now we know density =mass/volume
So, we calculate the mass by putting the value of volume and density
$\therefore \;\rho (density) = \;\dfrac{m}{{\pi {r^2}h}}$
$ \Rightarrow \;m = \pi {r^2}h\rho $
Where r is radius of cylinder and h is height.
Now, the force required to stop the rotation ${\rm{F}}\,{\rm{ = mg}}$
Where g as (acceleration due to gravity).
$\therefore {\rm{F}}\;{\rm{ = }}\pi {{\rm{r}}^2}h\rho g$
Hence the force required to stop the rotation${\rm{F}}\;{\rm{ = }}\pi {{\rm{r}}^2}h\rho g$,

so the correct option (D) none of these.

Note: Gravity acts on each fluid molecule. The force is always exerted as the centre of mass of the fluid molecule and direction is towards the centre of mass of the body exerting the force. Therefore the fluid body moves in the direction its net acceleration, dictated by the sum of the acceleration of the individual particle.
Pressure is a scalar quantity defined as force per unit area where the force acts in a direction perpendicular to the surface.