
A man has fallen into a ditch of width
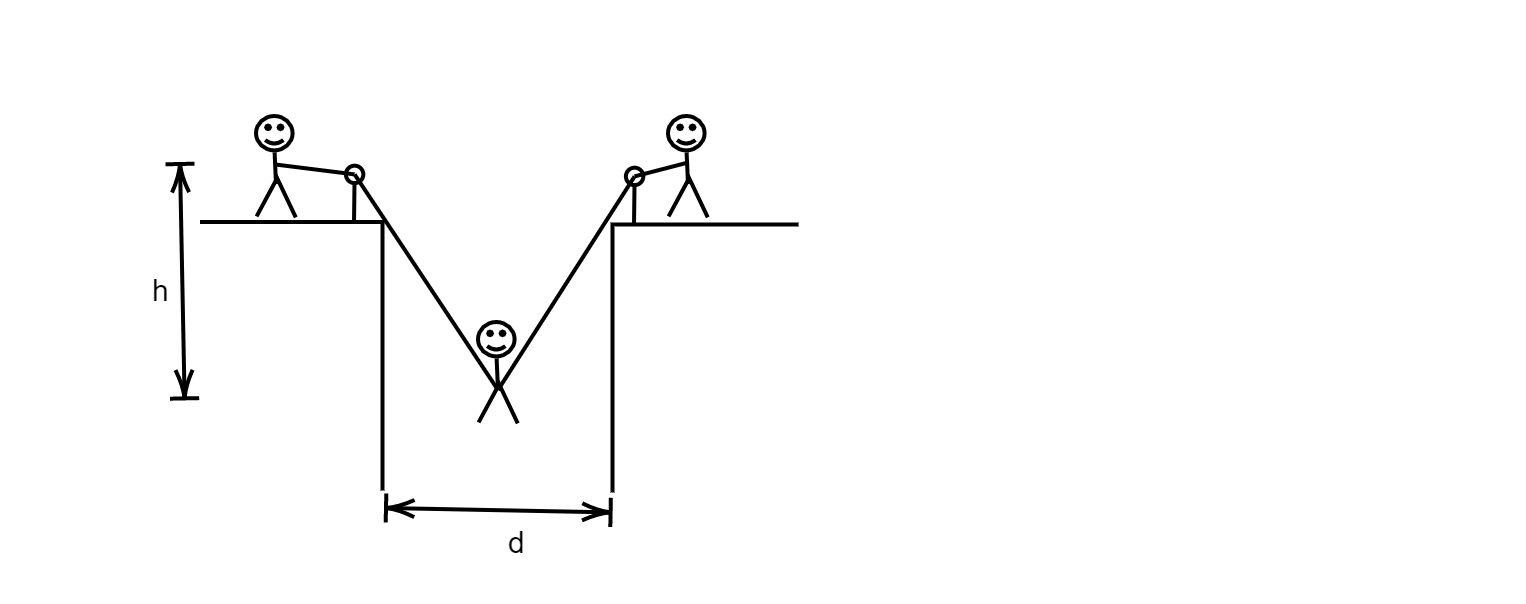

Answer
441.6k+ views
2 likes
Hint: To solve these types of questions, our first step should be to make a detailed free body diagram so that we can figure out how the forces are acting on the system. Next step would be to form equations of forces and then solve them to get the required results.
Complete answer:
Let us first draw the free body diagram for better understanding and assume that the rope makes an angle
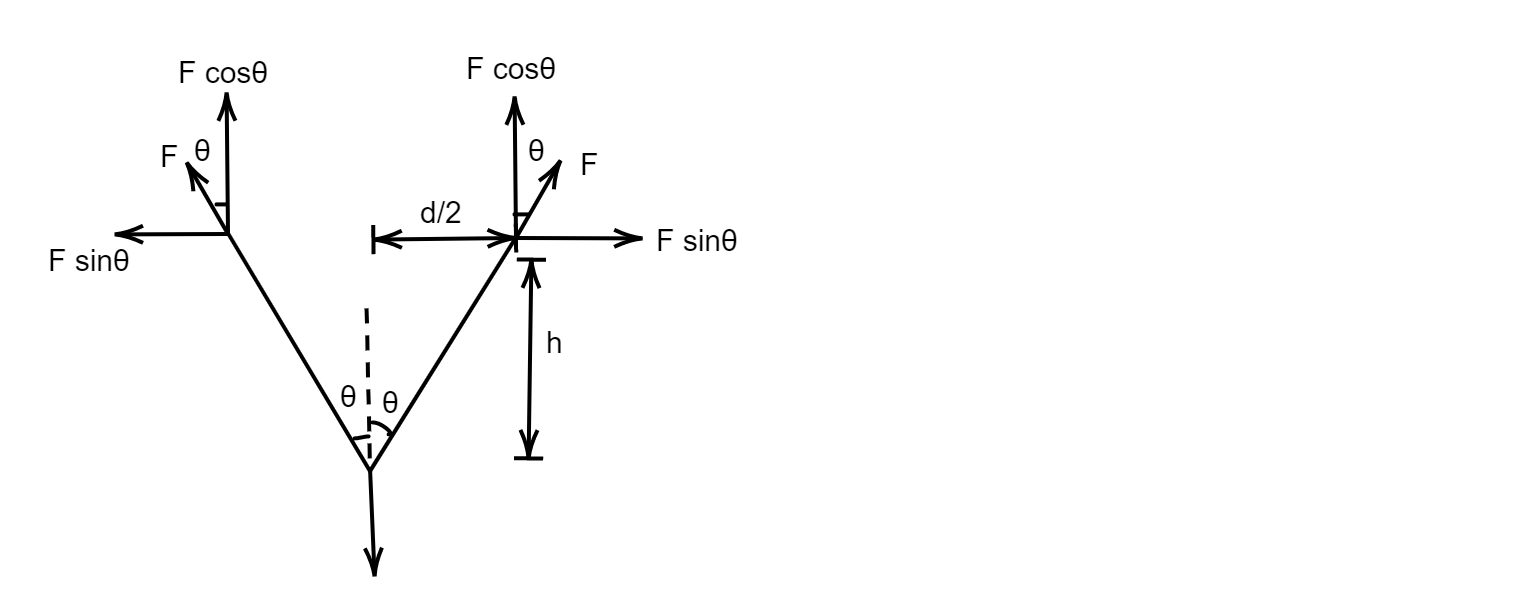
On observing the free body diagram, we can conclude that:
As the man’s friends pull him up, the angle that the rope makes with the vertical will increase and thus
We know that
Substituting this value in equation of force, we get:
Hence, the force exerted by each of the friends increases as the man moves up.
Note:
An infinite amount of force would have to be applied to make the depth of the ditch zero, because force is inversely proportional to zero, hence if depth of the ditch decreases, i.e., as the man moves up, the force also increases and it would be practically impossible to make the horizontally straight by pulling the rope.
Complete answer:
Let us first draw the free body diagram for better understanding and assume that the rope makes an angle

On observing the free body diagram, we can conclude that:
As the man’s friends pull him up, the angle that the rope makes with the vertical will increase and thus
We know that
Substituting this value in equation of force, we get:
Hence, the force exerted by each of the friends increases as the man moves up.
Note:
An infinite amount of force would have to be applied to make the depth of the ditch zero, because force is inversely proportional to zero, hence if depth of the ditch decreases, i.e., as the man moves up, the force also increases and it would be practically impossible to make the horizontally straight by pulling the rope.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




