
A man in a boat rowing away a lighthouse
Answer
434.5k+ views
Hint: We have to find the speed of the boat, to calculate speed we need to find the distance that boat travelled from the lighthouse. We find the distance by using the angle elevation and tangent formula. After finding the distance we can find the speed of the boat in metres per minute.
Formula used:
Complete step-by-step answer:
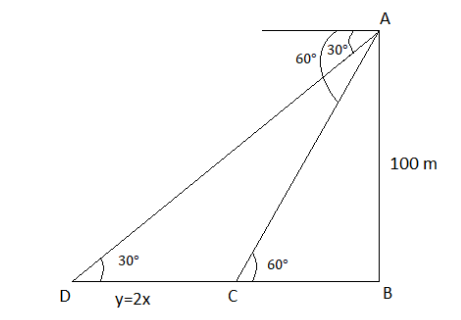
We construct the question as a diagram to understand easily.
Consider
Here
Let
Now we are going to find the distance between
From
Consider
By using the tangent formula in
Substituting the value of
Let us solve this for
Now we are going to find the distance between
From
Consider
By using the tangent formula in
Substituting the value of
Let us solve this for
Consider,
Substituting the values of
Since,
Let us solve this for
Note: The term angle of elevation denotes the angle from the horizontal upward to an object. An observer’s line of sight would be above the horizontal. In this question we may go wrong on finding the speed of the boat and we will be careful on substituting the values. Given is already in required unit values. So we don’t change the unit values for speed and distance.
Formula used:
Complete step-by-step answer:

We construct the question as a diagram to understand easily.
Consider
Here
Let
Now we are going to find the distance between
From
Consider
By using the tangent formula in
Substituting the value of
Let us solve this for
Now we are going to find the distance between
From
Consider
By using the tangent formula in
Substituting the value of
Let us solve this for
Consider,
Substituting the values of
Since,
Let us solve this for
Note: The term angle of elevation denotes the angle from the horizontal upward to an object. An observer’s line of sight would be above the horizontal. In this question we may go wrong on finding the speed of the boat and we will be careful on substituting the values. Given is already in required unit values. So we don’t change the unit values for speed and distance.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE




