Question

A man is standing on the 8 m long shadow of a 6 m long pole. If the length of his shadow is 2.4 m, what is the height of the man?
(A) 1.8 m
(B) 1.6 m
(C) 1.4 m
(D) 2.0 m

Answer 1

Hint: Make the geometric construction of the height of the pole and the shadow coasted by the pole using triangle. For each 1 m height of both pole and man, the coasted shadow should be the same. Then use the properties of triangles to determine the height of the man.

Complete step by step answer:
We can draw the geometric representation of the height of the pole and shadow of the pole as shown in the figure below.

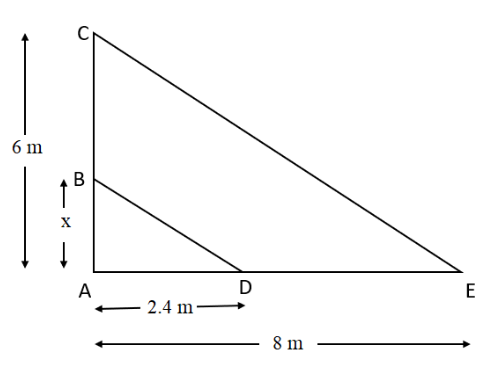
In the above figure, AC is the height of the pole, AB is the height of the man, AE is the length of the shadow coasted by the pole and AD is the length of the shadow coasted by the man.
From the geometry of the above figure, we can write,
\[ \Rightarrow\dfrac{{{\text{AC}}}}{{{\text{AE}}}} = \dfrac{{\text{x}}}{{{\text{AD}}}}\]
Here, x is the height of the man.
Substitute 6 m for AC, 8 m for AE, and 2.4 m for AD in the above equation.
\[ \Rightarrow\dfrac{{{\text{6}}\,m}}{{{\text{8}}\,m}} = \dfrac{{\text{x}}}{{{\text{2}}{\text{.4}}\,m}}\]
\[ \Rightarrow x = \dfrac{{\left( {2.4\,m} \right)\left( {6\,m} \right)}}{{8\,m}}\]
\[ \Rightarrow\therefore x = 1.8\,m\]
So, the correct answer is option (A).

Note:You can also solve this question by determining how much shadow is coasted by 1 m height of the pole. Then multiplying this value by the length of the shadow coasted by the man you can get the height of the man.