Question

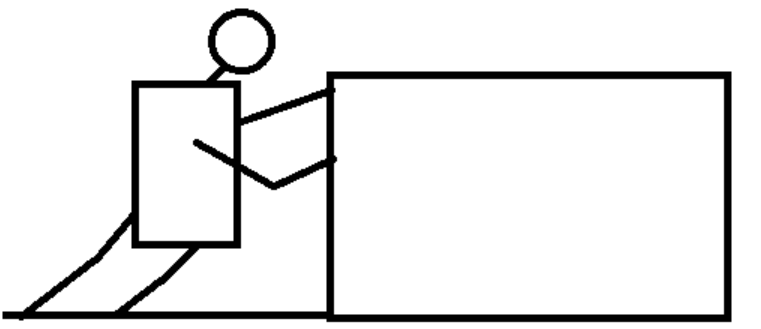
A man of mass $75kg$ is pushing a heavy box on a flat floor. The coefficient of kinetic and static friction between the floor and the box is $0.20$, and the coefficient of static friction between the man's shoes and the floor is $0.80$. If the man pushes horizontally to see the figure, what is the maximum mass ( in kg ) of the box he can move?

A. $300kg$
B. $600kg$
C. $900kg$
D. None of these

Answer 1

Hint: We can solve the problem with the concept of friction. Friction is a resisting force that acts between the two surfaces that are sliding or trying to slide across each other. Friction always acts in the opposite direction of the moving body. It is the result of the electromagnetic attraction between charged particles in two touching surfaces.

Complete step-by-step solution:
The amount of friction is nearly independent of the area of contact. The man can only push as much weight as the friction force provides. Friction is proportional to the weight means when a body feels heavy, it experiences more friction to move. Thus the ratio of friction force to the weight of the body ( Normal force) is constant. This constant ratio is called the coefficient of friction and the symbol of this is $\mu$. This is the dimensionless constant. Mathematically it is represented as;
$\mu ={\displaystyle \frac{f}{N}}$, where $N$ is normal force $f$ if the friction force.
The box will only move when the force applied $F$ on it is greater than the friction force $f_B$ available for it. So,
 $F⩾f_B={\mu }_B{m}_{B}g=0.2m_{B}g$, $m_B$ is the mass of the box.
If the man is moving the box, then there is no slipping off the shoes of the man on the floor. So friction on the man $f_m$ will be static.
$f_m=F$ but $f_m⩽{\mu }_{s}75g\Rightarrow F⩽{\mu }_{s}75g$, so we will get
= $0.2mg⩽F⩽{\mu }_{s}75g$
$\Rightarrow 0.2mg⩽{\mu }_{s}75g$
$\Rightarrow m⩽{\displaystyle \frac{0.8}{0.2}}\times 75=m⩽300kg$
If the man pushes horizontally the box, $300kg$ is the maximum mass ( in kg ) of the box he can move. Option is correct A.

Note: There are two types of friction, static friction, and kinetic friction. The one operates between the objects which are in rest and the second one acts between the objects in motion. Static friction is more than the kinetic friction. In nature, there are no completely frictionless environments.