Question

A man of mass m standing on a plank of mass M which is placed on a smooth horizontal surface, is initially at rest. The man suddenly starts running on the plank with a speed of \[vm/s\] with respect to the ground. Find the speed of the plank with respect to the ground.

Answer 1

Hint: Conservation of linear momentum can be used to find the velocity of plank with respect to the ground. According to this initial momentum will be equal to the final momentum. In this case initial momentum will be zero since there is no motion at that time. So, we can equate zero to the final momentum. Therefore, we can find the speed of the plank.

Complete step by step answer:
This man-plank system is a linear momentum conserved system or centre of mass conserved system and there are no external forces since it is a smooth surface.

We can do it with the conservation of linear momentum concept.

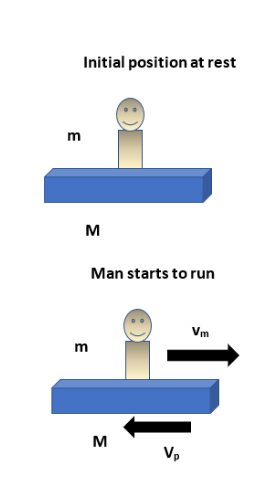
According to the question m is the mass of man and M is the mass of plank. The man is running on the plank with a speed of \[{{v}_{m}}\] with respect to the ground. Assume the \[{{V}_{p}}\] is the speed of the plank with respect to the ground. \[V\]is the velocity of the man with respect to the plank.

\[V={{v}_{m}}-{{V}_{p}}\]

Velocity of the man with respect to the ground will be,

\[{{v}_{m}}={{V}_{p}}+V\]……….(1)

Now we can apply the conservation of momentum to the system.
According to this concept, initial momentum will be equal to final momentum.
Initially the momentum is zero, since there is no movement to the system.

Hence the final momentum will be equal to zero.

\[m{{v}_{m}}+M{{V}_{p}}=0\]………..(2)
We can put equation (1) in equation (2) to get a simpler form.

\[m(V+{{V}_{p}})+M{{V}_{p}}=0\]
So, the velocity of the plank with respect to the ground is given below.

\[M{{V}_{p}}+m{{V}_{p}}=-mV\]
\[(m+M){{V}_{p}}=-mV\]
\[{{V}_{p}}=\dfrac{-mV}{(m+M)}\]

Negative sign indicates the movement of the plank towards the opposite direction of the man.

Additional information:
Momentum is conserved in all types of collisions.
Both momentum and kinetic energy is conserved in elastic collision.
Momentum is conserved but kinetic energy is not conserved in non-perfect inelastic collisions. In this case the objects won’t stick together after the collision.

Momentum is conserved but kinetic energy is not conserved in inelastic collision. In this case the objects stick together after the collision. Therefore, their velocities will be the same.

Note: In the question we can see the smooth horizontal surface term. It means, there are no external forces like frictional force that will resist free motion. If you are doing conservation of the centre of mass, you should use displacement instead of velocity.