
A massless conical flask filled with a liquid is kept on a table in a vacuum. The force exerted by the liquid on the base of the flask is

A.
B.
C.
D. The force exerted by the liquid on the walls of the flask is

Answer
501.6k+ views
2 likes
Hint: As a first step, you could go for finding the force exerted by the liquid on the walls of the given flask. We will then get the resultant of this force only in the upward direction as all its horizontal components on either side of the flask get cancelled. This force when subtracted from the force exerted by the liquid at the base will give you
Complete step-by-step answer:
We are given a massless conical flask filled with a liquid. Also the force exerted by the liquid on the base of the flask is taken as
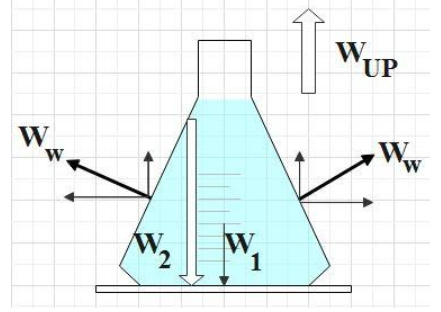
Recall that, by Pascal’s law, the pressure for a liquid at rest is the same at all points provided they are at the same height. So, there definitely exists a pressure that is exerted by the liquid at the walls of the conical flask. Let us consider a pressure exerted at corresponding points on either side of the conical flask and let us represent the force responsible for it by
The force
Since
Also, from (1) we have,
Where, ‘
Hence, the correct options for this question are option B and option D.
Note: If you are worried about not considering the atmospheric pressure that is exerted at the surface of the liquid, remember that the system under consideration is at vacuum which has no atmosphere. Also note that, we are also excluding weight (mg) of the conical flask exerted against gravity. This is because we are given a massless flask in the question, hence the weight is negligible.
Complete step-by-step answer:
We are given a massless conical flask filled with a liquid. Also the force exerted by the liquid on the base of the flask is taken as

Recall that, by Pascal’s law, the pressure for a liquid at rest is the same at all points provided they are at the same height. So, there definitely exists a pressure that is exerted by the liquid at the walls of the conical flask. Let us consider a pressure exerted at corresponding points on either side of the conical flask and let us represent the force responsible for it by
The force
Since
Also, from (1) we have,
Where, ‘
Hence, the correct options for this question are option B and option D.
Note: If you are worried about not considering the atmospheric pressure that is exerted at the surface of the liquid, remember that the system under consideration is at vacuum which has no atmosphere. Also note that, we are also excluding weight (mg) of the conical flask exerted against gravity. This is because we are given a massless flask in the question, hence the weight is negligible.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




