
A metallic block of density 5 gm/
A.
B.
C.
D.
Answer
485.1k+ views
1 likes
Hint: To find the apparent weight of the given block, we will first find the actual weight of the block using the given density of the block. We will then substitute this value in the formula for the apparent weight of a body. We know, that buoyant force is the force exerted on an object that is fully or partially submerged in liquid. This force is equal to the weight of the liquid dispersed by the body.
Formula Used: -
Complete step-by-step solution:
We know that the relative density of the material is the ratio of the density of the material over a reference material such as water for liquid and solid, and air for gas
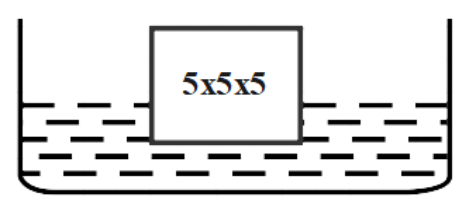
Let us first write the given parameters,
The actual weight say W is given by,
We know, that weight is terms of density is given by,
From (1), (2) and (3)
We get,
We have not substituted the value of g as in option it is still unsubstituted.
Now,
We know the formula to for the apparent weight is given by,
After substituting the values,
We get,
On solving the equation, we get,
Rearrange the terms,
We know that,
Let,
Therefore,
Therefore, the correct answer is option D.
Note: Apparent weight is a property of an object which determines how heavy the given object is. The difference between actual weight and apparent weight is that true weight is the mass of the object times the gravity and apparent weight is the sum of net force when submerged in liquid or other cases like standing in an elevator or floating in the lower of the earth. The concept of apparent weight is mostly used to find the apparent weight of a body in an elevator. It is also useful when applied in fluidization.
Formula Used: -
Complete step-by-step solution:
We know that the relative density of the material is the ratio of the density of the material over a reference material such as water for liquid and solid, and air for gas

Let us first write the given parameters,
The actual weight say W is given by,
We know, that weight is terms of density is given by,
From (1), (2) and (3)
We get,
We have not substituted the value of g as in option it is still unsubstituted.
Now,
We know the formula to for the apparent weight is given by,
After substituting the values,
We get,
On solving the equation, we get,
Rearrange the terms,
We know that,
Let,
Therefore,
Therefore, the correct answer is option D.
Note: Apparent weight is a property of an object which determines how heavy the given object is. The difference between actual weight and apparent weight is that true weight is the mass of the object times the gravity and apparent weight is the sum of net force when submerged in liquid or other cases like standing in an elevator or floating in the lower of the earth. The concept of apparent weight is mostly used to find the apparent weight of a body in an elevator. It is also useful when applied in fluidization.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




