
A milk container is made of metal sheet in the shape of frustum of a cone whose volume is
Answer
530.4k+ views
Hint: At first use the volume formula
Complete step-by-step answer:
In the question we are given a milk container which is in the shape of a frustum of a cone. We have to find the total surface area of the cone so as to get the cost of the metal sheet to cover the container.
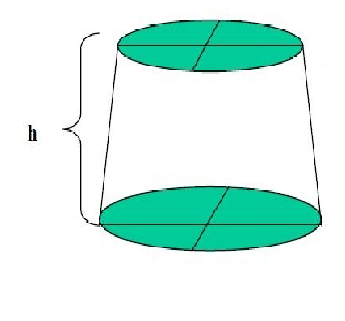
The data or the dimensions of the frustum of the cone given are radii of its lower and upper circular surfaces are 8cm and 20cm respectively. The volume is already given as
To find the surface area we are given the formula with opening on the smaller side of base ‘r’ of frustum,
Where R, r are the radius of larger and smaller circular bases respectively and h is height.
The formula of volume is,
Where R, r are the radius of larger and smaller circular bases respectively and h is height.
The volume given is
Now it can be written as,
Here
So,
Which can be written as,
Which can be further solved as,
So,
Hence the height of the frustum of the cone is 16cm.
Now, we have to get the value of surface area of frustum with an opening on the circular base of radius ‘r’,
Here
So, the surface area is,
Which can be calculated as,
By doing calculations carefully we can write it as,
Hence, the area of metal sheet required is
Now for the costing it was given that for
So, the total cost is
Hence the cost of metal sheet is Rs. 4224.
Note: Students might get confused in this problem whether to include
Complete step-by-step answer:
In the question we are given a milk container which is in the shape of a frustum of a cone. We have to find the total surface area of the cone so as to get the cost of the metal sheet to cover the container.

The data or the dimensions of the frustum of the cone given are radii of its lower and upper circular surfaces are 8cm and 20cm respectively. The volume is already given as
To find the surface area we are given the formula with opening on the smaller side of base ‘r’ of frustum,
Where R, r are the radius of larger and smaller circular bases respectively and h is height.
The formula of volume is,
Where R, r are the radius of larger and smaller circular bases respectively and h is height.
The volume given is
Now it can be written as,
Here
So,
Which can be written as,
Which can be further solved as,
So,
Hence the height of the frustum of the cone is 16cm.
Now, we have to get the value of surface area of frustum with an opening on the circular base of radius ‘r’,
Here
So, the surface area is,
Which can be calculated as,
By doing calculations carefully we can write it as,
Hence, the area of metal sheet required is
Now for the costing it was given that for
So, the total cost is
Hence the cost of metal sheet is Rs. 4224.
Note: Students might get confused in this problem whether to include
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




