Question

A painter sets a ladder up to reach the bottom of a second storey window 16 feet above the ground. The base of the ladder is 12 feet from the house. While the painter mixes the paint a neighbor’s dog bumps the ladder which moves the base 2 feet farther away from the house. How far up the side of the house does the ladder reach?

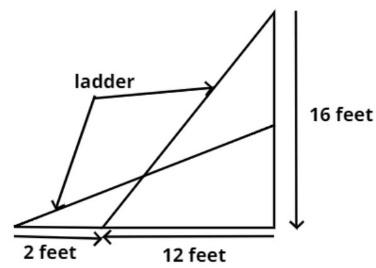
Answer 1

Hint – In this particular question first of all mark all the points in the given figure. It will help us to find out what is asked then later on in the solution use the concept of the Pythagoras’ theorem so use these concepts to reach the solution of the question.

Complete step-by-step answer:

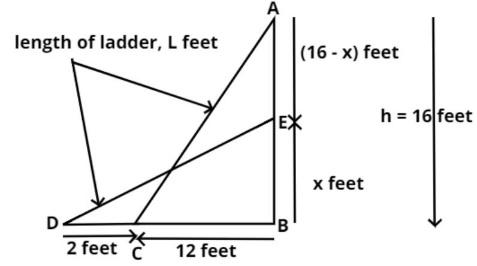
Let the length of the ladder be L feet as shown in the above figure.
Therefore, AC = DE = L feet (see figure).
Let AB be the two storey building as shown in the figure.
Therefore, AB = 16 feet (see figure).
Let BC be the base of the ladder from the building.
Therefore, BC = 12 feet (see figure).
Now during the mixing of the paint a dog bumps and it moves the base 2 feet farther away from the house.
Therefore, CD = 2 feet (see figure).
Now let the top of the ladder from the ground point of the building be at x feet (see figure).
Therefore, EB = x feet (see figure).
So the distance AE = (16 – x) feet (see figure).
Now in triangle ABC apply Pythagoras’ theorem we have,
$ \Rightarrow {\left( {{\text{hypotenuse}}} \right)^2} = {\left( {{\text{perpendicular}}} \right)^2} + {\left( {{\text{base}}} \right)^2}$
Now substitute the values we have,
$ \Rightarrow {\left( {\text{L}} \right)^2} = {\left( {{\text{AB}}} \right)^2} + {\left( {{\text{BC}}} \right)^2}$
$ \Rightarrow {\left( {\text{L}} \right)^2} = {\left( {{\text{16}}} \right)^2} + {\left( {{\text{12}}} \right)^2} = 256 + 144 = 400 = {\left( {20} \right)^2}$
So the length of the ladder = 20 feet.
Now in triangle EBD apply Pythagoras’ theorem we have,
$ \Rightarrow {\left( {{\text{hypotenuse}}} \right)^2} = {\left( {{\text{perpendicular}}} \right)^2} + {\left( {{\text{base}}} \right)^2}$
Now substitute the values we have,
$ \Rightarrow {\left( {\text{L}} \right)^2} = {\left( {{\text{EB}}} \right)^2} + {\left( {{\text{BD}}} \right)^2}$
$ \Rightarrow {\left( {\text{L}} \right)^2} = {\left( {{\text{EB}}} \right)^2} + {\left( {{\text{BC}} + {\text{CD}}} \right)^2}$
$ \Rightarrow {\left( {20} \right)^2} = {\left( {\text{x}} \right)^2} + {\left( {{\text{12 + 2}}} \right)^2} = {x^2} + {\left( {14} \right)^2}$
$ \Rightarrow {x^2} = 400 - 196 = 204$
Now take square root on both sides we have,
$ \Rightarrow x = \sqrt {204} = 14.2828$ Feet.
So the top of the ladder from the ground point of the building = 14.2828 feet.
So this is the required answer.

Note – Whenever we face such types of questions first find out the length of the ladder using Pythagoras theorem as above, then using this length of the ladder apply the Pythagoras’ theorem in the lower triangle and evaluate the length does the ladder reach from the bottom, we will get the required answer.