
A parachutist is descending vertically and makes angles of elevation of
Answer
519.3k+ views
Hint: consider the maximum height as AB and draw the angle of elevations at two different points and apply
Complete step-by-step answer:
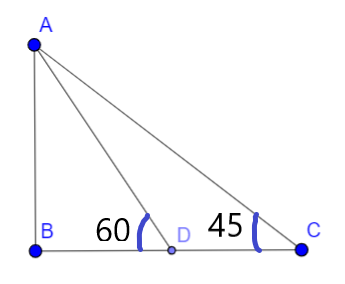
Given, the angle of elevations are
Let the maximum height be AB and BD be x.
In the right angled triangle ABC
In the right angled triangle ABD
Substitute
So, the maximum height from which he falls is AB=
Note: The angle of elevation is the angle between the horizontal line from the observer and the line of sight to an object that is above the horizontal line. As the person moves from one point to another angle of elevation varies. If we move closer to the object the angle of elevation increases and vice versa.
Complete step-by-step answer:

Given, the angle of elevations are
Let the maximum height be AB and BD be x.
In the right angled triangle ABC
In the right angled triangle ABD
Substitute
So, the maximum height from which he falls is AB=
Note: The angle of elevation is the angle between the horizontal line from the observer and the line of sight to an object that is above the horizontal line. As the person moves from one point to another angle of elevation varies. If we move closer to the object the angle of elevation increases and vice versa.
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




