Question

A parallel beam of rays is incident on a convergent lens with a focal length of $40cm$. Where should a diverging lens with a focal length of $15cm$ can be placed for the beam of rays to remain parallel after passing through the two lenses? Draw a ray diagram.

Answer 1

Hint: The parallel beam of the rays is incident on a convergent lens and then passes through a diverging lens then the rays become parallel to the principal axis if the rays fall on the focus of the convergent lens.

Complete step by step solution:

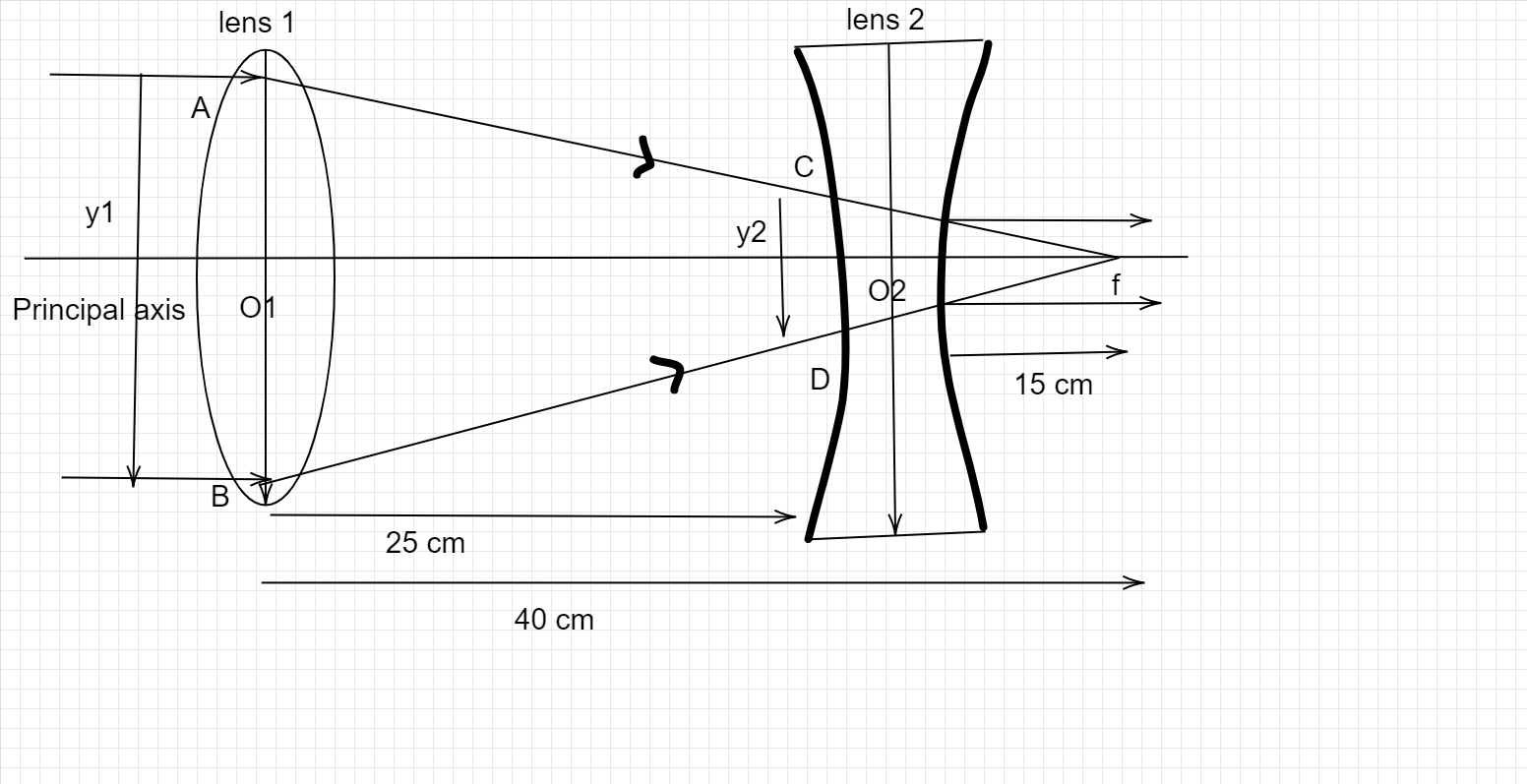
Here, $f$ is the focus of both the convergent and divergent lens.
$O1$ and $O2$ are the centers of both the lenses.
$A,B,C,D$ are the points where the rays of light are incident on the lens.
$Y1$ and $Y2$ are the vertical distance between the points where the rays meet the lens.
When the rays which are travelling parallel to the convergent lens meet at the focus but before that, they will meet at a divergent lens. As given in the question the rays must be parallel after passing through the divergent lens.
The focus of both the lenses should be the same for the rays to be parallel at the end.
Let the distance between the two lenses be ${{x}}$
$\Rightarrow 40{{cm }} = 15{{cm}} + {{x}}$
$ \Rightarrow {{x}} = 40{{ cm - 15 cm = }}25{{cm}}$

Additional information:
A converging lens is called a convex lens. In a convex lens, the light rays enter parallel to the horizontal axis of the lens and converge at a single point on the opposite side. A diverging lens is a concave lens in which light rays that enter parallel to the horizontal axis bend away from its axis.

Note: As the lenses are concave and convex lenses when two rays come from infinity, they will pass through the focus of both the lenses and then travel parallel to the horizontal axis. The focal length of the convergent lens will be a sum of the focal length of the divergent lens and the distance between them.