Question

A particle is projected with a velocity u making an angle $\theta$ with the horizontal. T any instant, its velocity v is at right angles to its initial velocity u; then v is:
$
  {\text{A}}{\text{. }}u\cos \theta \\
  {\text{B}}{\text{. }}u\tan \theta \\
  {\text{C}}{\text{. }}u\cot \theta \\
  {\text{D}}{\text{. }}u\sec \theta \\
$

Answer 1

Hint: We can resolve the initial velocity and velocity at a certain point on trajectory into their horizontal and vertical components. Then by using basic trigonometric relations between the components, we can find the required answer.

Step by step solution:
When an object is thrown with some initial velocity and is allowed to fall freely under the effect of gravity of earth, then the object follows a curved path. The object is known as a projectile while this type of motion exhibited by an object under gravity is called the projectile motion.

When a projectile is projected with some initial velocity u then as it moves along its path, its velocity keeps changing and is not fixed at u.
In this question, we have a particle which is projected with a velocity u and makes an angle
$\theta $ with the horizontal. Diagrammatically, we have the following scenario.

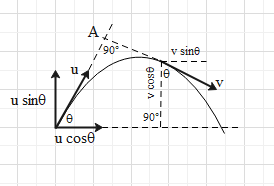
We can resolve the initial velocity into its horizontal and vertical components. We are given that at every point of trajectory, the velocity v of the particle perpendicular to its initial velocity which is represented in the diagram by reproducing the directions of u and v meeting at 90$^\circ $
 at point A.

Like u, we can resolve the components of v as shown. Now we see that horizontal components of u and v must be equal. Therefore, we can write the following expression for the particle.

${\text{v sin}}\theta = u\cos \theta $

Rearranging the terms, we get the required answer to be

$
  {\text{v}} = u\dfrac{{\cos \theta }}{{\sin \theta }} \\
   \Rightarrow {\text{v}} = u\cot \theta \\
$

Hence, the correct answer is option C

Note: The velocity of the projectile keeps changing along its trajectory. As the projectile rises above the ground, its velocity keeps on decreasing. At the highest point v=0. As the projectile starts falling, v starts increasing but final velocity is zero as projectile comes to rest when it completes its trajectory.