
A particle on a stretched string supporting a travelling wave, takes minimum time
Answer
499.8k+ views
Hint: Try to understand that the wave is produced by particles vibrating about their mean positions. Remember that the time period defines the amount of time the particle takes to execute one vibration(cycle) and is inversely proportional to the frequency of vibration. Intuitively recall the relation that relates frequency and wavelength to the wave speed by looking at the units they are expressed in.
Formula used:
Time taken for a particle to move from mean position back to mean position =
Frequency of vibration
Wave speed
Complete step by step answer:
We have a particle vibrating on a stretched string. Such a vibration carries a wave. This is a case of simple harmonic motion.
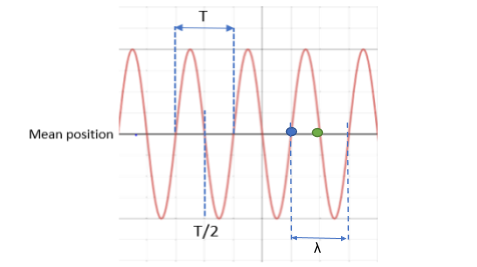
If we take T to be the total time period of vibration, the vibrating particle reaches the mean position back at
The question says that the particle takes
a)Therefore, our frequency of vibration f is found to be:
b)The distance between two particles is given as
With the help of the diagram, we can deduce that the wavelength of the wave produced as a result of these vibrations is two times the distance between consecutive vibrating particles i.e.,
Wavelength
c)The wave speed can be expressed in terms of wavelength and wave frequency as follows:
Wave Speed
Note:
Sometimes the question is tweaked by providing the time taken for the particle to vibrate from the mean to the extreme position. In that case, remember that the vibrating particle will take
Formula used:
Time taken for a particle to move from mean position back to mean position =
Frequency of vibration
Wave speed
Complete step by step answer:
We have a particle vibrating on a stretched string. Such a vibration carries a wave. This is a case of simple harmonic motion.

If we take T to be the total time period of vibration, the vibrating particle reaches the mean position back at
The question says that the particle takes
a)Therefore, our frequency of vibration f is found to be:
b)The distance between two particles is given as
With the help of the diagram, we can deduce that the wavelength of the wave produced as a result of these vibrations is two times the distance between consecutive vibrating particles i.e.,
Wavelength
c)The wave speed can be expressed in terms of wavelength and wave frequency as follows:
Wave Speed
Note:
Sometimes the question is tweaked by providing the time taken for the particle to vibrate from the mean to the extreme position. In that case, remember that the vibrating particle will take
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




