
A pulley of radius 2m is rotated about its axis by a force
(A). More than 3 but less than 6
(B). More than 6 but less than 9
(C). More than 9
(D). Less than 3
Answer
513k+ views
1 likes
Hint: Here we are applying force to rotate a pulley. Before stopping it will do some rotations. Since we have already given the force that we are applying, the moment of inertia of the pulley and the radius of the pulley, we can apply the same laws of linear motion to the rotational motion.
Formula used:
Complete step by step answer:
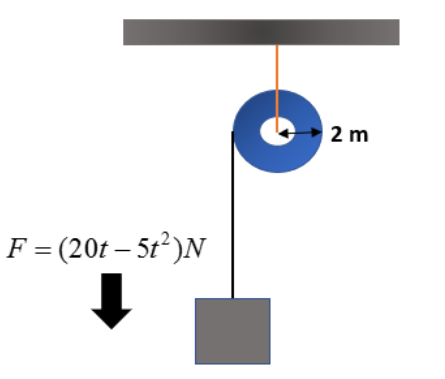
Consider a pulley of radius 2 m. Its moment of inertia about the axis is already given in the question. It is about
Torque can find out from the force and radius of the pulley.
Next, we can find out the angular acceleration from the torque.
As we know angular acceleration is the derivative of angular velocity. Thus, we can write as,
So that we have to integrate this, to find out the angular velocity.
Here we are going to integrate from 0 to t.
Now we can assign the limits to the equation.
Angular velocity will be zero at a point. So we can find out the time at which the velocity will be zero.
The angular velocity will be zero within 6 seconds.
Now we can find out the angular displacement from 0 to 6 seconds.
We can assign the angular velocity equation here.
We can put the limits to the equation.
So, the number of revolutions will be
Therefore the revolutions will be less than 6. So the correct option is A.
Note: Do not forget to make the angular velocity as zero. Then only we can find out the time period required for the stopping of the pulley. During this time it will move 36-radians angular displacement. So do not stop the calculation there. Because we have calculated the total angular displacement. We have to calculate the number of rotations from there. It may not be an integer value. That’s why the options are given in a range.
Formula used:
Complete step by step answer:

Consider a pulley of radius 2 m. Its moment of inertia about the axis is already given in the question. It is about
Torque can find out from the force and radius of the pulley.
Next, we can find out the angular acceleration from the torque.
As we know angular acceleration is the derivative of angular velocity. Thus, we can write as,
So that we have to integrate this, to find out the angular velocity.
Here we are going to integrate from 0 to t.
Now we can assign the limits to the equation.
Angular velocity will be zero at a point. So we can find out the time at which the velocity will be zero.
The angular velocity will be zero within 6 seconds.
Now we can find out the angular displacement from 0 to 6 seconds.
We can assign the angular velocity equation here.
We can put the limits to the equation.
So, the number of revolutions will be
Therefore the revolutions will be less than 6. So the correct option is A.
Note: Do not forget to make the angular velocity as zero. Then only we can find out the time period required for the stopping of the pulley. During this time it will move 36-radians angular displacement. So do not stop the calculation there. Because we have calculated the total angular displacement. We have to calculate the number of rotations from there. It may not be an integer value. That’s why the options are given in a range.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




