
A quadratic polynomial has no zero. Its graph…..
A) Touches X-axis at any point
B) Intersects X-axis at two distinct points
C) Does not intersect X-axis at any points
D) Is in any half-plane of the X-axis.
Answer
485.1k+ views
1 likes
Hint: A quadratic polynomial is a polynomial of degree 2. An equation involving a quadratic polynomial is a quadratic equation. The standard form is
with a, b, and c being constants or numerical coefficients, and x is an unknown variable. One absolute rule is that the first constant "a" cannot be a zero.
Complete step-by-step solution:
The x-intercepts are the points at which the parabola crosses the x-axis. If they exist, the x-intercepts represent the zeros, or roots, of the quadratic function.
Now, in the question, it is mentioned that a given quadratic polynomial has no zero.
Let us assume that the quadratic polynomial is the form
We also know that the roots of the above quadratic polynomial are
X=
We also know that for a quadratic equation if x-intercept exist it means that the given equation has roots or also it can be concluded that the graph of the polynomial crosses the x- axis.
But, from our question, it is said that the quadratic polynomial has no zero, which means there exists no x for which the graph intersects the x-axis.
In the diagram below we can see an example of a parabola which is the graph of a quadratic polynomial, it is given in the question that it doesn’t have a zero which implies that the quadratic equation had no roots and hence it doesn’t intersect the x-axis. As the roots of the polynomial lie on the x-axis.
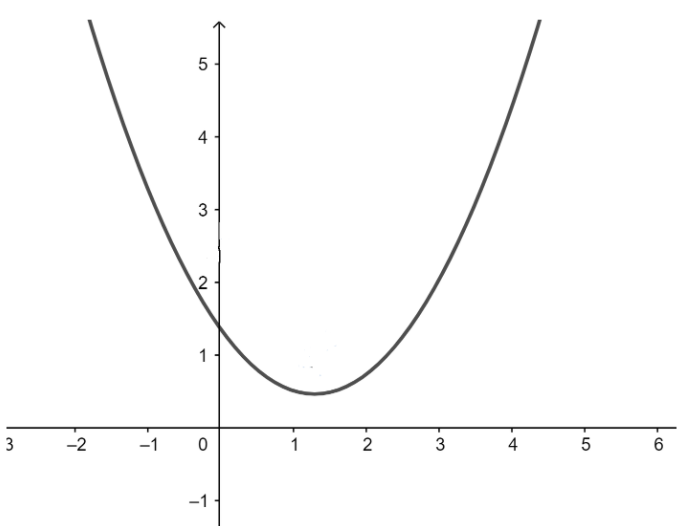
In the diagram below the parabola touches the x-axis, from which we can conclude that the parabola would have two equal roots.
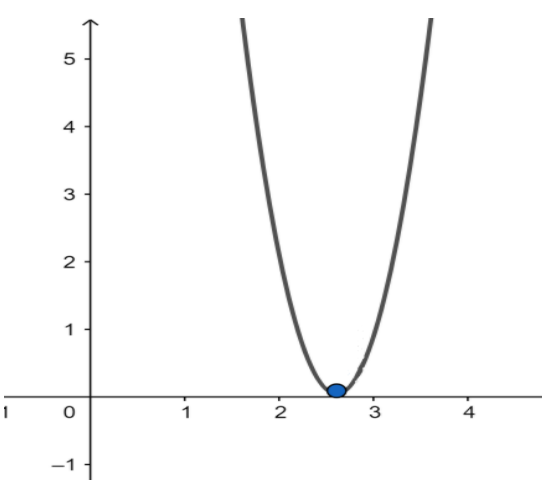
In option A it is given that the graph touches the x-axis at one point, which would be false because if the graph intersects the x-axis at one point then y=0 will be zero for some x.
Hence option C is correct which is the graph doesn’t intersect the x-axis at any point.
Note: Students should read the question properly or they might get confused with what is actually given. A quadratic polynomial has at most two real roots so the maximum no of times the graph will intersect the x-axis is two and the minimum would be zero when the equation has no roots.
with a, b, and c being constants or numerical coefficients, and x is an unknown variable. One absolute rule is that the first constant "a" cannot be a zero.
Complete step-by-step solution:
The x-intercepts are the points at which the parabola crosses the x-axis. If they exist, the x-intercepts represent the zeros, or roots, of the quadratic function.
Now, in the question, it is mentioned that a given quadratic polynomial has no zero.
Let us assume that the quadratic polynomial is the form
We also know that the roots of the above quadratic polynomial are
X=
We also know that for a quadratic equation if x-intercept exist it means that the given equation has roots or also it can be concluded that the graph of the polynomial crosses the x- axis.
But, from our question, it is said that the quadratic polynomial has no zero, which means there exists no x for which the graph intersects the x-axis.
In the diagram below we can see an example of a parabola which is the graph of a quadratic polynomial, it is given in the question that it doesn’t have a zero which implies that the quadratic equation had no roots and hence it doesn’t intersect the x-axis. As the roots of the polynomial lie on the x-axis.

In the diagram below the parabola touches the x-axis, from which we can conclude that the parabola would have two equal roots.

In option A it is given that the graph touches the x-axis at one point, which would be false because if the graph intersects the x-axis at one point then y=0 will be zero for some x.
Hence option C is correct which is the graph doesn’t intersect the x-axis at any point.
Note: Students should read the question properly or they might get confused with what is actually given. A quadratic polynomial has at most two real roots so the maximum no of times the graph will intersect the x-axis is two and the minimum would be zero when the equation has no roots.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




