
A quadrilateral ABCD is drawn to circumscribe a circle. Prove that
Answer
515.4k+ views
Hint: We will suppose a circle with center as ‘O’ which is circumscribed by quadrilateral ABCD and the quadrilateral touches the circle at four points and then we will use the property of tangents of a circle that the lengths of tangents drawn from the external points are equal.
Complete step-by-step answer:
We have been given a quadrilateral ABCD circumscribing the circle then we have to prove that
Let us suppose a circle with center ‘O’ which is circumscribed by the quadrilateral ABCD and touches the circle at point P, Q, R and S.
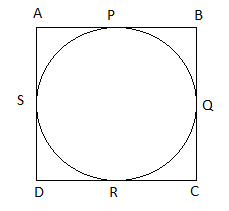
As we know that the length of tangents drawn from the external points are equal.
Therefore,
AP=AS…..(1)
BP=BQ…..(2)
DR=DS……(3)
CR=CQ…..(4)
Now adding equation (1), (2), (3) and (4) and we get as follows:
On rearranging the terms we get as follows:
Since we can see from the figure that,
AB=AP+BP
BC=BQ+CQ
CD=CR+DR
AD=AS+SD
Using these values, we get as follows:
AB+CD=AD+BC
Therefore, the required expression is proved.
Note: Remember the point that the length of a tangent from an external point to the circle is equal. In this question without a diagram we are unable to prove the given expression. So first of all draw the diagram according to the question.
Complete step-by-step answer:
We have been given a quadrilateral ABCD circumscribing the circle then we have to prove that
Let us suppose a circle with center ‘O’ which is circumscribed by the quadrilateral ABCD and touches the circle at point P, Q, R and S.

As we know that the length of tangents drawn from the external points are equal.
Therefore,
AP=AS…..(1)
BP=BQ…..(2)
DR=DS……(3)
CR=CQ…..(4)
Now adding equation (1), (2), (3) and (4) and we get as follows:
On rearranging the terms we get as follows:
Since we can see from the figure that,
AB=AP+BP
BC=BQ+CQ
CD=CR+DR
AD=AS+SD
Using these values, we get as follows:
AB+CD=AD+BC
Therefore, the required expression is proved.
Note: Remember the point that the length of a tangent from an external point to the circle is equal. In this question without a diagram we are unable to prove the given expression. So first of all draw the diagram according to the question.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




